Module 7. Mass transfer

Lesson 31

PROBLEMS BASED ON HEAT AND MASS TRANSFER

Q. 1. Evaluate the diffusion coefficient of CO₂ in air at 20°C and atmospheric pressure.

The following data is given:

CO2	3.996	190
Air	3.617	97

 Π = 1.044

Solution:

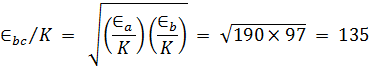
T = 20+273 = 293°K

P = 1 atm

 Π = 1.044

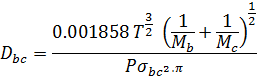
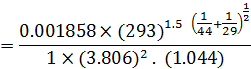
= 0.147 cm²/ sec.

Q. 2. Estimate the diffusion rate of water from the bottom of a test tube 1.5 cm in diameter and 15 cm long into dry atmospheric air at 25°C.

Assume D = 0.256 cm²/s

Solution:

Air will be saturated at the bottom of the test tube at x = 0 then the partial pressure of water vapour at x = 0 will be saturation pressure of water vapour at 25°C.

at                x =L =15cm (length of the tube)

At the top, air does not contain any water vapour

       P_w2 = 0

        P_{a2 =} P_t = 1.0303.

Now using the following equation

D= 0.256 × 0.36 m²/hr,   T = 25+273      M_w =18

P_t = 1.0303 ata= 1.0303 ×10⁴ kg/m² ,  x₁ = 0 ,  x₂ = 0.15 m

G = 848

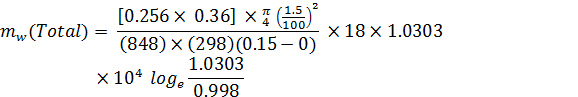
= 1.086 × 10^-6 kg/hr.

Q. 3. Calculate the rate of diffusion of water vapor from a pool of water at the bottom of a well 6 metre in height to dry air flowing over the top of the well. Assume the entire system is at 25°C and 1 atm. If the well diameter is 3 meters, then find out the total weight of water diffused per hour from the surface of the water in the well.

Assume D = 0.256 cm²/s

Solution:

D = 0.256 x 0.36 m²/hr,     x₁ = 0,      x₂ = 6 m

P_a1 = P_t – P_w1 =1.0303 – 0.0323 = 0.998

P_a2 = P_t – P_w2 =1.0303 – 0.00 = 1.0303

A = 1 m²,   M_w = 18,    G = 848,   T = 298.

Using the following equation

= 1.125 ×10^-4 kg/m²-hr.

Total  mass flow = mass flow rate × well surface area

= 1.125 × 10^-4 × π/4 (3)² = 7.94 × 10^-4 kg/hr.