Module 3.  Pressure measuring devices

Lesson 7

DIFFERENTIAL MANOMETERS

7.1  Introduction

Differential manometers are used to measure the difference of pressures between two points in a pipe or in two different pipes. There are two types of differential manometers.

1. U-tube upright differential manometer

2. U-tube inverted differential manometer

7.2  U-tube Upright Differential Manometer

It is used to measure pressure difference at two points in a pipe or between two pipes at different levels.

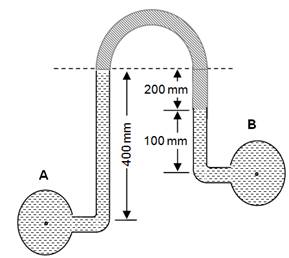
Case 1 - U-tube upright differential manometer connected at two points in a pipe at same level

The construction and arrangement of a manometer connected at two different points, A and B, of a pipe is shown in figure 7.1.

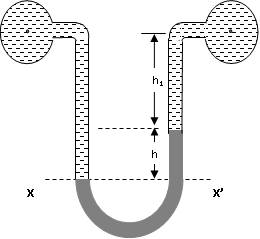
Fig. 7.1 U-tube upright differential manometer

Let,         

ρ₁ = density of liquid flowing in the pipeline

ρ₂ = density of manometer liquid (assume mercury)

S = Specific gravity of liquid for which pressure has to be determined

S₁ = Specific gravity of manometer liquid

h_A be the pressure in terms of height of fluid in the pipe at point A

h_B be the pressure in terms of height of fluid in the pipe at point B

h is the distance of mercury level in the right limb from the datum line XX’

h₁ is the height of manometer liquid level in the right limb from the centre of pipe at point B.

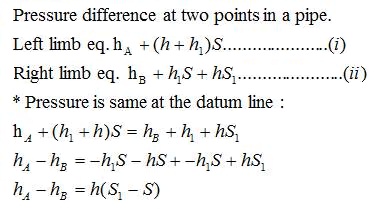
Case 2 - U-tube upright differential manometer connected between two pipes at different levels and carrying different fluids

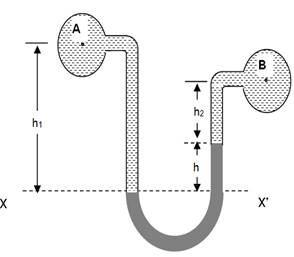
Fig. 7.2 Vertical differential manometer (pressure difference between two pipes)

Let,         

S₁ = Specific gravity of liquid in pipe A

S₂ = Specific gravity of liquid in pipe B

S = Specific gravity of manometer liquid

h_A be the pressure head in terms of height of fluid in the pipe at point A

h_B be the pressure head in terms of height of fluid in the pipe at point B

h is the distance of mercury level in the right limb from the datum line XX’

h₁ is the height of manometer liquid level in the left limb from the from the datum line XX’

h₂ is the height of manometer liquid level in the right limb from the from the centre of pipe at point B.

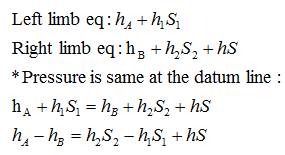
7.2 U-tube Inverted Differential Manometer

In such types of manometers light fluids for e.g. oil is used as manometer fluid. In the previous derivation, the term (h×S) is added, but here in the left and right limb equations, it is necessary to subtract (h×S) term.

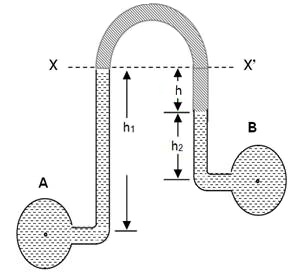
Fig. 7.3 Inverted differential manometer

Let,         

S₁ = Specific gravity of liquid in pipe A

S₂ = Specific gravity of liquid in pipe B

S = Specific gravity of manometer liquid

h_A be the pressure head in terms of height of fluid in the pipe at point A

h_B be the pressure head in terms of height of fluid in the pipe at point B

h is the distance of manometer liquid level in the right limb from the datum line XX’

h₁ is the height of manometer liquid level in the left limb from the from the datum line XX’

h₂ is the height of manometer liquid level in the right limb from the from the centre of pipe at point B

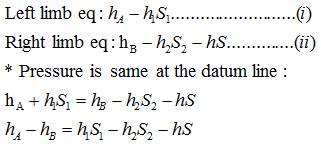
7.4  Numerical

Q1. A U-tube monometer is used to measure the pressure of oil (specific gravity 0.85) flowing in a pipe line. It’s left end connected to pipe and right limb is open to the atmosphere. The centre of pipe is 100 mm below the level of mercury in the right limb. If the difference of mercury level in the two line is 160 mm, then determine the head and pressure.

Solution:

h = 13.6 × 160 × 10^-3 – 0.85 × 60 × 10^-3

                           = 2176 × 10^-3 – 51.00 × 10^-3

                           = (2176-51) × 10^-3

                           = 2125 × 10^-3

                           = 2.125 m

                        P = ρgh

                           = 1000 × 9.81 × 2.125

                           = 20846.25 N/m²

Q2. A U-tube monometer containing mercury was used to find the negative pressure in the pipe. The right limb was open to the atmosphere; find the vacuum pressure in pipe if the difference of mercury level in two pipes is 100 mm and height of water in the left limb from the centre of pipe was found to 40 mm.

Solution:

h = - (h₁S₁+ h₂S₂)

= - (40×1+100×13.6) × 10^-3

= - (40+1360) × 10^-3

= -1400 × 10^-3

= -1.4 m

P = ρgh

= 13734 N/m²

Q3. A simple U-tube manometer containing mercury is connected to a pipe in which an oil of specific gravity 0.80 is flowing. The pressure in the pipe is vacuum. The other end of the manometer is open to atmosphere, find the vacuum pressure in pipe if the difference of mercury level in two limbs is 200 mm and height of oil in left end from the centre of pipe is 150 mm below.

Solution.

h = h₂S₂- h₁S₁

               = (200 × 13.6 – 150 × 0.8) × 10^-3

               = (2720-120) × 10^-3

               = 2600 × 10^-3

               = 2.6 m                    

            P = ρgh

               = 800 × 9.81 × 2.6

               = 20404.8 N/m²

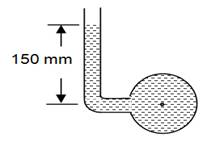
Q 1: A piezometer was connected to a pipe to measure the pressure of water. The rise of water level in piezometer was 150 mm. Calculate the pressure of water. [Ans = 1471.5 N/m²]

Q 2: Pressure in a pipe carrying oil (Specific Gravity 0.92) is 20 kN/m². A piezometer is connected to the pipe. Find the rise of liquid level in the piezometer. [Ans = 2.21 m]

Q 3: A U-tube manometer is connected to a pipe for measuring the pressure of oil (Specific gravity 0.92) flowing in the pipeline. Mercury (Specific gravity 13.6) is used as manometer liquid and the difference of mercury level in the two limbs is 200 mm. The centre of pipe is 80 mm below the level of mercury in the right limb. Determine the pressure in the pipeline and the head.  [Ans: 23.55 kN/m²]

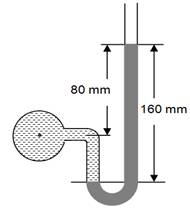
Q 4: The pressure of water in a domestic supply line was measured using manometer shown in the figure. Derive the equation for head and determine the pressure.   [Ans: h= 1.99 m, p = 19.52 kN/m²]

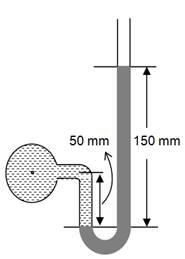
Q 5: Negative pressure in a water pipe was measured using U-tube manometer containing mercury (Specific gravity 13.6). The left limb was connected to the water pipe and right limb was exposed to the atmosphere. Height of water upto centre of pipe in the left limb was 30 mm. Difference between the mercury levels in the two limbs was 50 mm. Determine the vacuum pressure in the pipe and also derive the equation used. [Ans: h= -0.710 m, p = -6.965 kN/m²]

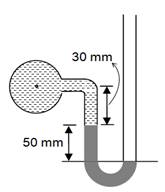
Q 6: A vertical column micro manometer is connected to a pipe containing oil of specific gravity 0.92. The ratio of area of reservoir to that of vertical column is 150. Calculate the oil pressure in the pipe. [Ans: h= 7.98 m, p = 78.28 kN/m²]

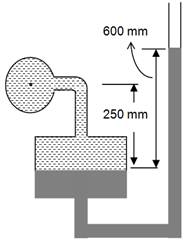
Q 7: Difference in pressure in pipe at two points A and B was measured using a differential manometer. The specific gravity of oil in the pipe is 0.85. If the difference in the mercury level in the two limbs is 200 mm, calculate the pressure difference. [Ans: h= 2.55 m, p = 25.01 kN/m²]

Q 8: Pressure difference in a water pipe at two points A and B was measured using a differential manometer. If the difference in the mercury level in the two limbs is 50 mm, calculate the pressure difference between two points. [Ans: h= 0.63 m, p = 6.18 kN/m²]

Q 9: A U-tube differential manometer is connected to two pipes at A and B. Pipe A Contains oil of Specific Gravity 0.92 and pipe B is carrying water. If the pressure at point A is 125 kN/m² find the pressure at point B. [Ans: h= -3.098 m, p = 30.39 kN/m²]

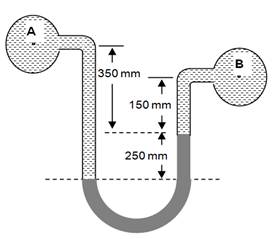
Q 10: An inverted tube differential manometer having an oil of specific gravity 0.9 is connected to two different pipes carrying water under pressure. Determine the pressure in the pipe B. The pressure in pipe A is 2 m of water. [Ans: h= 1.88 m, p = 18.44 kN/m²]