Module 2.  A.C. series and parallel circuits

Lesson 9

STAR DELTA TRANSFORMATION

9.1  Transformations

Alternating voltage and current can be shown using phasor diagram. The problems can be analysed by using following two mathematical forms:

9.1.1  Rectangular form

Consider a voltage phasor V. The magnitude of the phasor is V and having θ angle from reference line OX.

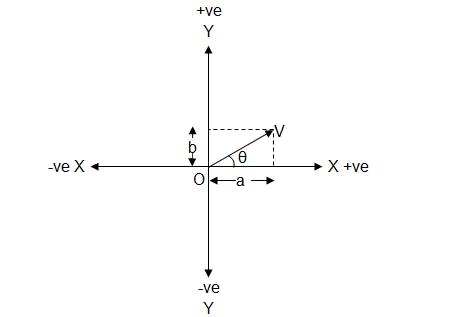
Fig. 9.1 Voltage phasor denoted by OV

Voltage phasor V = a + jb

Magnitude of phasor

9.1.2  Polar form

In polar form the phasor can be represented by the magnitude and the angle with the reference axis.

V = V∠ θ°

9.2  Conversion Methods

1. To convert Rectangular to polar form:

           Rectangular form V = a + jb

           Polar form = V ∠ θ°

2. To convert Polar to Rectangular form:

Rectangular form = V ∠ θ°

a = V cos θ

                         b = V sin θ

Polar form = a + jb

9.3  Numerical

Q.1 Convert 100 + j 50 to polar form:

            Magnitude =

             = 111.80

Phase angle =

                = 26.56°         

Polar form = 111.80∠ 26.56°

Q.2 Convert 100∠ 14.47° to rectangular form.

a = V cosθ

= 100 cos14.47°

= 96.82

b = V sin θ

=100 sin 14.47°

= 25

Rectangular form = 96.82 + j25

Q.3 Convert 200−j50 and −25−j20 into polar forms.

1. 200-j50 into polar

Magnitude =

    = 206.15

            Phase angle

     = −14.03°

Polar form = 206.15∠−14.03°

2. −25−j20 into polar

Magnitude =

             = 32.01

 Phase angle

       = 38.65°                          

Polar form = 32.01∠38.65°

9.4 Adding and Subtraction of Phasors

The rectangular form is the simplest method for addition or subtraction of Phasors. If the phasors are represented in polar form, they should be first converted to rectangular form and then addition or subtraction be carried out.

(i) Addition: For the addition of phasors in the rectangular form, the real components are added together and the complex numbers (j components) are added together. Consider two voltage phasors:

V₁ = a₁ + jb₁;    V₂ = a₂ + jb₂

Resultant Voltage       V = V₁ + V₂ = (a₁ + jb₁) + (a₂ + jb₂)

                                        = (a₁ + a₂) + j(b₁ + b₂)

Magnitude of resultant, V

Angle from OX-axis,

(ii)Subtraction is done similar to what was done in phasor addition

V = V₁ −V₂ = (a₁ + jb₁) − (a₂ + jb₂)

= (a₁ − a₂) + j(b₁ − b₂)

Magnitude of resultant voltage,            

Angle from OX-axis,  

 9.5  Multiplication and Division of Phasors

Multiplication and division of phasor is done in polar form as the method is simpler compared to what is done in rectangular form. Consider two phasor:

V₁ = a₁ + jb₁ = V₁∠θ₁

V₂ = a₂ + jb₂ = V₂∠θ₂

9.5.1  Multiplication

(i) Rectangular form,

V₁ × V₂ = (a₁ + jb₁) (a₂ + jb₂)

= a₁a₂ + ja₁b₂ + ja₂b₁ + j²b₁b₂

= (a₁a₂−b₁b₂) + j(a₁b₂ + a₂b₁)                     (as  j²=−1)

           Magnitude of resultant

Angle w.r.t. OX-axis, 

(ii) Polar form. To multiply the phasors that are in polar form, multiply their magnitudes and add the angles (algebraically).

V₁ × V₂ = V₁∠θ₁× V₂ ∠θ₂ = V₁V₂∠ θ₁+ θ₂

Multiplication of phasors becomes easier when they are expressed in polar form.

9.5.2  Division

(i)  Rectangular form,

(ii) Polar Form. To divide the phasors that are in polar form, the magnitude of phasors are divided and denominator angle is subtracted from the numerator angle.

9.6  Transformation  

9.6.1  Star to delta (Y/∆) transformation

In any electrical system a star Y connection may be replaced by an equivalent ∆-connected system. A 3-phase star system having voltage V_L and line current I_L may be replaced by a ∆-connected system having phase voltage V_L and phase current I_L/√3. Y-connected load having branch impedances each of Z∠∅ may be replaced by an equivalent ∆-connected load having phase impedance is 3Z∠∅ (Fig. 1.2).

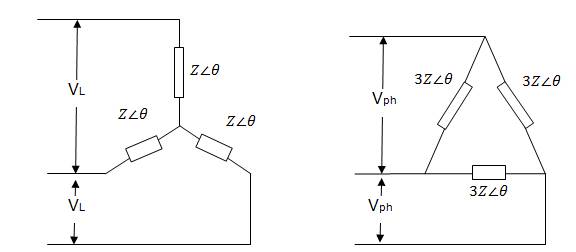
Fig. 9.2 Star to delta (Y/∆) transformation

For a balanced star-connected load, let

V_L = line voltage;

I_L = line current;

Z∠∅ = impedance per phase

Then for an equivalent ∆-connected system,

            Phase voltage V_ph = V_L

 Phase current I_ph = I_L / √3 

Z_ph = 3Z∠∅

Z_Y = V_L / (√3 I_L)

 9.6.2  Delta to star (∆/Y) transformation

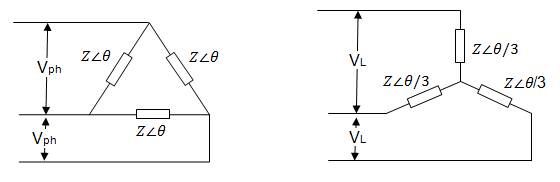
Fig. 9.3 Delta to star (∆/Y) transformation

Now, in the equivalent ∆-connected systems, the line voltages and currents must have the same values as in the Y-connected system, hence we must have

V_L= V_ph

I_ph = I_L / √3

Z_∆ = V_L / (I_L / √3) = √3 V_L/ I_L = 3Z_Y

Z_∆ ∠ ∅ = 3 Z_Y∠ ∅                                                                    (∵V_L/ I_L = 3Z_Y)

Z_∆ = 3 Z_Y or Z_Y = Z_∆ /3

Numerical

1. A 220 V 3 phase Y connected load is shown in following diagram. The phase sequence is RYB.  Calculate the line currents and neutral line current.

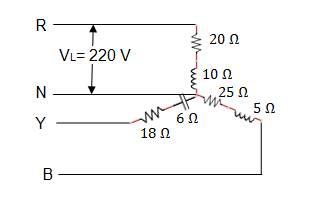
Z_R = (20 + j10) = 22.36∠26.56^o

Z_Y = (18 + j6) = 18.97∠18.43^o

Z_Y = (25 + j5) = 25.49∠11.30^o

Let line voltages with difference of phase angle 120^o

V_RN = 220∠0^o

V_YN = 220∠─120^o

V_BN = 220∠─240^o

Line currents:

I_R = V_RN/Z_R = =9.83∠─26.56^o = 8.79 ─ j4.39 A

I_Y = V_YN/Z_Y = =11.59∠─138.43^o = ─ 8.67 ─ j7.69 A

I_B = V_BN/Z_B = =8.63∠─251.3^o = ─ 2.76 + j8.17 A

 I_N = I_R + I_Y + I_B

= 8.79 ─ j4.39 ─ 8.67 ─ j7.69 ─ 2.76 + j8.17

I_N = ─ 2.64 + j4.87 = 5.53∠118.46^o A

2. Consider 75 ohm resistors are connected in star and then in delta.  If line voltage V_L = 440 V calculate line and phase current in star and delta system.

Solution

Star connection

Phase voltage V_ph = V_L/√3 = 440/√3 = 254.03 V

Phase current I_ph = V_ph / Z _ph  = 254.03/75 = 3.38 A

Line current I_L = Phase current I_ph = 3.38 A

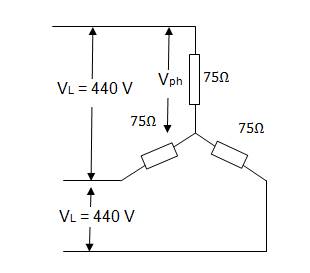
Delta connection

Phase voltage V_ph = Line voltage V_L  = 440 V

Phase current I_ph = V_ph / Z_ph = 440/75 = 5.86 A 

Line current I_L = I_ph X √3  = 5.86 X √3   = 10.14 A