Site pages
Current course
Participants
General
MODULE 1. FLUIDS MECHANICS
MODULE 2. PROPERTIES OF FLUIDS
MODULE 3. PRESSURE AND ITS MEASUREMENT
MODULE 4. PASCAL’S LAW
MODULE 5. PRESSURE FORCES ON PLANE AND CURVED SUR...
MODULE 6.
MODULE 7. BUOYANCY, METACENTRE AND METACENTRIC HEI...
MODULE 8. KINEMATICS OF FLUID FLOW
MODULE 9: CIRCULATION AND VORTICITY
MODULE 10.
MODULE 11.
MODULE 12, 13. FLUID DYNAMICS
MODULE 14.
MODULE 15. LAMINAR AND TURBULENT FLOW IN PIPES
MODULE 16. GENERAL EQUATION FOR HEAD LOSS-DARCY EQ...
MODULE 17.
MODULE 18. MAJOR AND MINOR HYDRAULIC LOSSES THROUG...
MODULE 19.
MODULE 20.
MODULE 21. DIMENSIONAL ANALYSIS AND SIMILITUDE
MODULE 22. INTRODUCTION TO FLUID MACHINERY
LESSON 4. PRESSURE
4.1 WHAT IS PRESSURE?
-
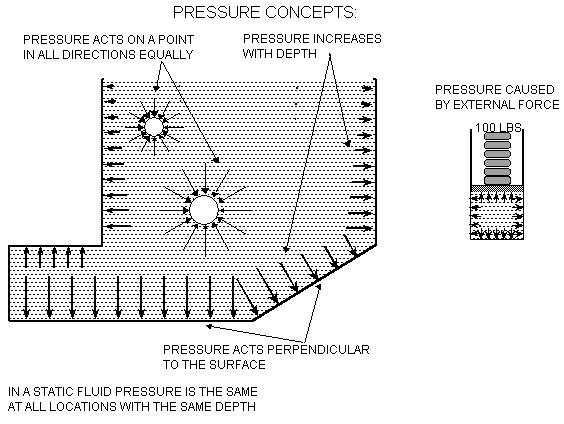
Pressure is the average of the normal forces acting at a point
-
Differences between normal forces are due to fluid motion
In this case, if the force vectors are equal in magnitude, then
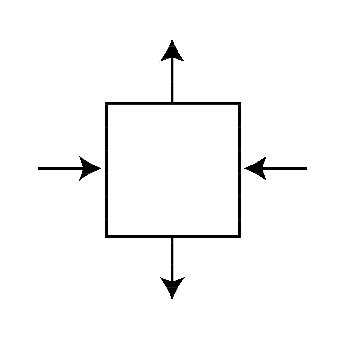
p = 0
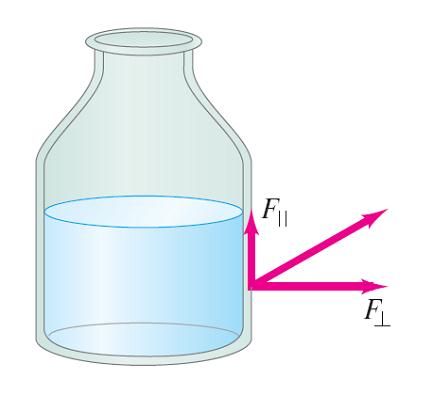
- The basic property of a static fluid is pressure.
- Pressure is defined as the amount of surface force exerted by a fluid on any boundary it is in contact with. It can be written as:
\[Pressure={{Force}\over{Area\;of\;which\;the\;force\;is\;applied}}\]
\[P={F\over A}\quad \]
Unit: N / m2 or Pascal (Pa).
(Also frequently used is bar, where 1 bar = 105 Pa).
4.2 Absolute Pressure, Gage Pressure, and Vacuum
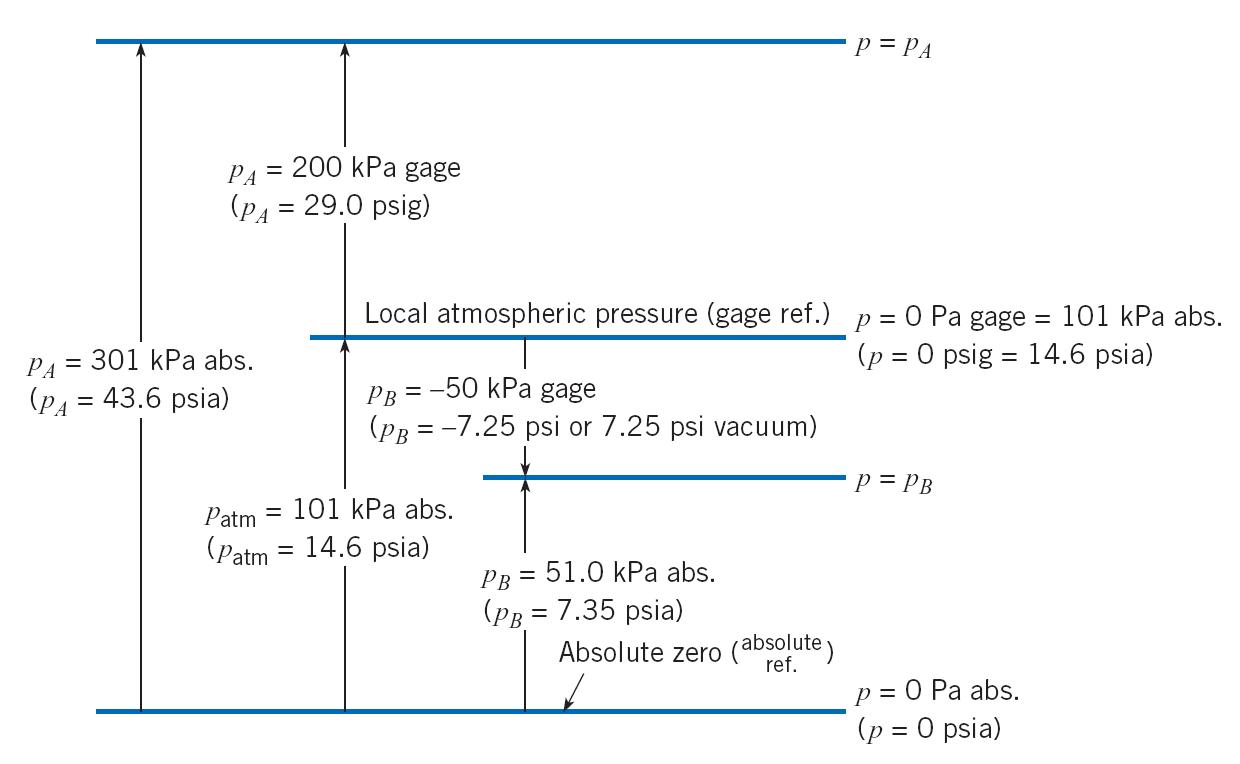
Pressure in a vacuum is p = 0.
Absolute pressure is referenced to perfect vacuum.
Gage pressure is referenced to another pressure, typically atmospheric pressure (most gages measure relative pressures).
Pressure measurements are generally indicated as being either absolute or gauge pressure.
is the pressure measured above or below the atmospheric pressure (i.e. taking the atmospheric as datum).
can be positive or negative.
A negative gauge pressure is also known as vacuum pressure.
uses absolute zero, which is the lowest possible pressure.
Therefore, an absolute pressure will always be positive.
refers to the prevailing pressure in the air around us.
It varies somewhat with changing weather conditions, and it decreases with increasing altitude.
At sea level, average atmospheric pressure is 101.3 kPa (abs), 14.7 psi (abs), or 1 atmosphere (1 bar = 1x105 Pa).
This is commonly referred to as ‘standard atmospheric pressure’.
A simple equation relating the two pressure measuring system can be written as
4.2.1 Gauge pressure
is the pressure measured above or below the atmospheric pressure (i.e. taking the atmospheric as datum).
can be positive or negative.
A negative gauge pressure is also known as vacuum pressure.
4.2.2 Absolute pressure
uses absolute zero, which is the lowest possible pressure.
Therefore, an absolute pressure will always be positive.
4.2.3 Atmospheric pressure
refers to the prevailing pressure in the air around us.
It varies somewhat with changing weather conditions, and it decreases with increasing altitude.
At sea level, average atmospheric pressure is 101.3 kPa (abs), 14.7 psi (abs), or 1 atmosphere (1 bar = 1x105 Pa).
This is commonly referred to as ‘standard atmospheric pressure’.
A simple equation relating the two pressure measuring system can be written as:
Pabs = Pgauge + Patm
Pressure acts uniformly in all directions on a small volume of fluid.
In a fluid confined by solid boundaries, pressure acts perpendicular to the boundary.
These principles, called Pascal’s Law,
A fluid at rest exerts pressure perpendicular to any surface that it contacts. There is no parallel component that would cause a fluid at rest to flow.
-
To find the variations of pressure with elevation, let’s consider a small cylindrical element of fluid of cross-sectional area A, and height (h = Z2 –Z1), surrounded by the same fluid of mass density, r.
-
The pressure at the bottom of the cylinder is P1 at level Z1, and at the top is P2 at level Z2. The fluid is at rest and in equilibrium so all the forces in the vertical direction sum to zero.
-
Force due to P1 (upward) = P1A
-
Force due to P2 (downward) = P2A
-
Force due to weight of element = mg = ρgA(Z2-Z1)
-
Taking the summation of forces (upward as positive);
(↑)ΣF=0
P1A – P2A - ρgA(Z2-Z1) = 0
P1 – P2 = ρg (Z2-Z1) = ρgh
or P2 – P1 = - ρg(Z2-Z1) = - ρgh
Thus, in any fluid under gravity,
an increase in elevation causes a decrease in pressure.
a decrease in elevation causes an increase in pressure.