Fluid Mechanics 3(2+1)

Lesson-16: FLOWNET

16.1.1 FLOW NETS FOR HOMOGENEOUS ISOTROPIC SYSTEMS

A flow net is a graphical solution to the equations of steady fluid flow. A flow net consists of two sets of lines which must always be orthogonal (perpendicular to each other): flow lines , which show the direction of groundwater flow, and equipotentials (lines of constant head), which show the distribution of potential energy.

Flow nets are usually constructed through trial-and-error sketching.

To construct a flow net:

1. make a two-dimensional scale drawing of the system under consideration (usually a profile, but may be a map view.)

2. determine or specify the boundary conditions, i.e., indicate/label the position of the water table, of any impermeable boundaries, of any points of known head or known pressure.

a. any surface of constant head (e.g., bottom of a flat-bottomed reservoir) is by definition an equipotential, and flow lines must meet it at right angles.

b. since flow cannot cross impermeable boundaries, the flow at such a boundary must be parallel to it, i.e., impermeable boundaries are flow lines, and equipotentials must meet them at right angles.

c. the water table is, by definition, the surface where P = 0; it can thus be an equipotential only if it is horizontal. At any point on the water table (no matter whether it is flat or sloping) h = z, where z is the elevation of the water table above the datum.

If there is no seepage percolating down to the water table, it can be considered a flow line. In the general case however (sloping water table, seepage across it), the water table is neither a flow line nor an equipotential, and flow lines will intersect it at an angle.

3. Once you have defined the boundary conditions, start trial sketching of flow lines and equipotentials, following the rules in step 2 above, and being sure that the flow lines and equipotentials always intersect at right angles.

Try to make the flow net consist of curvilinear "squares", i.e., the boxes in the flow net may have curving sides, but the midline lengths of the "square" should be approximately equal. (arrows inside square in diagram below) This is especially important if the flow net is to be used for calculations of groundwater discharge.

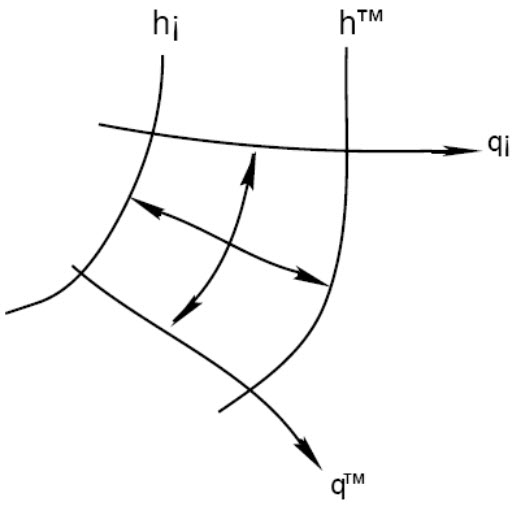
Keep sketching and refining until you have a good set of "squares" which satisfies the boundary conditions.

4. Determine the head at the left-most and right-most equipotentials and subtract them to get ∆h, the total head difference across the net. Now determine N_d , the number of potential drops (i.e.,squares) between these two equipotentials. The value of  each potential drop is thus:

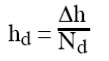
Knowing this, you can label each equipotential with its correct value of h.

5. To determine pore pressure at any point on an equipotential h, simply measure the elevation, z, of the point above the datum. Then the pressure is given by:

p = (h – z)γ where γ is the specific weight of water.