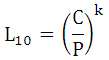Machine Design 3(2+1)

LESSON 28 SELECTION OF ANTIFRICTION BEARINGS

Reliability is the ratio of number of bearings that complete L million revolutions to the total number of bearings tested. Therefore, by definition, rated life (L₁₀) corresponds to 90% reliability of the bearing. Depending upon the type of application, bearings with reliability greater than 90% or less than 90% may also be required. Relation between bearing life and reliability is as follows:

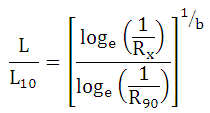
where,             L₁₀       = rated life of the bearing (life corresponding to 90% reliability)

                        L          = life of the bearing corresponding to x % reliability

R₉₀       = reliability of 90% (0.9)

R_x         = reliability of x %

Using this relation, for given rated life of bearing, life of bearing corresponding to a reliability of x % (L) can be calculated.

28.2          Static Load Carrying Capacity

Static load carrying capacity (C₀) of a bearing is defined as the static load corresponding to a total permanent deformation of balls and races, at the most heavily stressed point of contact, equal to 0.0001 of the ball diameter.

The bearing is subjected to some static load, when the shaft is stationary. This leads to plastic deformation in the balls and races. This deformation increases with increase in the static load. It has been established that a total permanent deformation of 0.0001 of ball diameter, at the most heavily stressed point of contact, can be tolerated without affecting operational properties of the bearing.

Different formulae have been developed for calculation of static load carrying capacity. However for selection of bearings, use of these formulae is not necessary; as the values of static load carrying capacity are directly given in manufacturer’s catalogue.

28.3          Dynamic Load Carrying Capacity

Dynamic load carrying capacity (C) is defined as the constant stationary radial load (in case of radial bearings) or constant axial load (in case of thrust bearings), which a group of apparently identical bearings, with stationary outer ring can endure for a rated life of one million revolutions with only 10 % failures.

Various relations have been developed for calculating dynamic load carrying capacity also, but again its value for different bearings is available in manufacturer’s catalogue. Table 28.1 gives basic dimensions and load capacities of different types of bearings.

28.4          Equivalent Bearing Load

In many applications, bearings are subjected to both radial and axial loads and are sometimes required to operate with stationary inner race and rotating outer race.

The equivalent dynamic load of a bearing is defined as the constant radial load in radial bearings or trust load in trust bearings, which if applied to the bearing, would give same life as attained by the bearing under actual conditions of loading. It is given by,

where,             V         = race rotation factor = 1       if inner race rotates

 = 1.2   if outer race rotates

X         = radial load factor

Y         = thrust load factor

Values of X and Y are available in manufacturer’s catalogue. Table 28.2 gives values of X and Y for deep groove ball bearings.

 Table 28.1 Dimensions & Load Carrying Capacities of Ball Bearings

Bearing No.	Bore (mm)	Outside Diameter (mm)	Width (mm)	Load Carrying Capacity (kN)
				Single Row Deep Groove		Single Row Angular Contact		Double Row Angular Contact		Self-aligning
				C0	C	C0	C	C0	C	C0	C
6200 6300	10	30 35	9 11	2.24 3.60	4 6.3	----- -----	----- -----	4.55 -----	7.35 -----	1.8 -----	5.7 -----
6201 6301	12	32 37	10 12	3.00 4.30	5.4 7.65	----- -----	----- -----	5.6 -----	8.3 -----	2 3	5.85 9.15
6202 6302	15	35 42	11 13	3.55 5.20	6.1 8.8	3.75 -----	6.30 -----	5.6 9.3	8.3 14	2.16 3.35	6 9.3
6203 6303 6403	17	40 47 62	12 14 17	4.40 6.30 11.00	7.5 10.6 18	4.75 7.2 -----	7.8 11.6 -----	8.15 12.9 -----	11.6 19.3 -----	2.8 4.15 -----	7.65 11.2 -----
6204 6304 6404	20	47 52 72	14 14 19	6.55 7.65 15.60	10 12.5 24	6.55 8.3 -----	10.4 13.7 -----	11 14 -----	16 19.3 -----	3.9 5.5 ------	9.8 14 -----
6205 6305 6405	25	52 62 80	15 17 21	7.10 10.40 19.00	11 16.6 28	7.8 12.5 -----	11.6 19.3 -----	13.7 20 -----	17.3 26.5 -----	4.25 7.65 -----	9.8 19 -----
6206 6306 6406	30	62 72 90	16 19 23	10.00 14.60 23.20	15.3 22 33.5	11.2 17 -----	16 24.5 -----	20.4 27.5 -----	25 35.5 -----	5.6 10.2 -----	12 24.5 -----
6207 6307 6407	35	72 80 100	17 21 25	13.70 17.60 30.50	20 26 43	15.3 20.4 -----	21.2 28.5 -----	28 36 -----	34 45 -----	8 13.2 ------	17 30.5 -----
6208 6308 6408	40	80 90 110	18 23 27	16 22 37.5	22.8 32 50	19 25.5 -----	25 35.5 -----	32.5 45.5 -----	39 55 -----	9.15 16 -----	17.6 35.5 -----
6209 6309 6409	45	85 100 120	19 25 29	18.3 30 44	25.5 41.5 60	21.6 34 -----	28 45.5 -----	37.5 56 -----	41.5 67 -----	10.2 19.6 -----	18 42.5 -----
6210 6310 6410	50	90 110 130	20 27 31	21.2 35.5 50	27.5 48 68	23.6 40.5 -----	29 53 -----	43 73.5 -----	47.5 81.5 -----	10.8 24 -----	18 50 -----
6211 6311 6411	55	100 120 140	21 29 33	26 42.5 60	34 56 78	30 47.5 -----	36.5 62 -----	49 80 -----	53 88 -----	12.7 28.5 -----	20.8 58.5 -----
6212 6312 6412	60	110 130 150	22 31 35	32 48 67	40.5 64 85	36.5 55 -----	44 71 -----	63 96.5 -----	65.5 102 -----	16 33.5 -----	26.5 68 -----
6213 6313 6413	65	120 140 160	23 33 37	35.5 55 76.5	44 72 93	43 63 -----	50 80 -----	69.5 112 -----	69.5 118 -----	20.4 39 -----	34 75 -----
6214 6314 6414	70	125 150 180	24 35 42	39 63 102	48 81.5 112	47.5 73.5 -----	54 90 -----	71 129 -----	69.5 137 -----	21.6 45 -----	34.5 85 -----
6215 6315 6415	75	130 160 190	25 37 45	42.5 72 110	52 90 120	50 81.5 -----	56 98 -----	80 140 -----	76.5 143 -----	22.4 52 -----	34.5 95 -----
6216 6316 6416	80	140 170 200	26 39 48	45.5 80 120	57 96.5 127	57 91.5 -----	63 106 -----	96.5 160 ------	93 163 -----	25 58.5 -----	38 106 -----
6217 6317 6417	85	150 180 210	28 41 52	55 88 132	65.5 104 134	65.5 102 -----	71 114 -----	100 180 -----	106 180 -----	30 62 -----	45.5 110 -----
6218 6318 6418	90	160 190 225	30 43 54	63 98 146	75 112 146	76.5 114 -----	83 122 ------	127 ----- -----	118 ----- -----	36 69.5 -----	55 118 -----

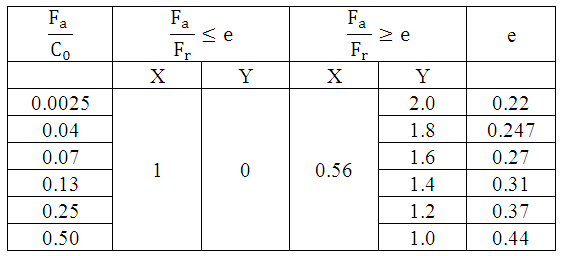
Table 28.2 Values of Radial Load Factor (X) and Thrust Load Factor (Y) for Deep Groove Ball Bearings

28.5          Load Life Relationship

Relationship between bearing life, dynamic load carrying capacity and equivalent dynamic load, is given by,

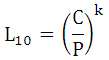
where,             L₁₀       = rated bearing life

C         = dynamic load carrying capacity

P          = equivalent dynamic load

k          = load life exponent    = 3       for ball bearings

= 10/3  for roller bearings

Also,

where,             L_10h      = rated bearing life in hours

N         = speed of rotation in r.p.m.

28.6          Selection of Bearings

Following steps are generally followed in selection of antifriction bearings:

1. Determine radial and axial forces (F_r and F_a respectively) acting on the shaft.
2. Calculate the diameter of the shaft.
3. Select suitable type of the bearing from manufacturer’s catalogue.

Following guidelines can be used for selecting ball bearings:

Type of Ball Bearing	Fa/ Fr
Single Row Deep Groove	0.5 – 0.8
Double Row Deep Groove	0.8 – 1.5
Angular Contact	1.5 – 2.0
Self Aligned	0.2 – 0.5

 

Select the lowest series of the selected category, depending upon the shaft diameter. Note the value of static load carrying capacity, C₀. Refer table 28.1.

4. Select value of race rotation factor, V.

5. Determine values of radial and thrust load factors (X and Y) corresponding to the calculated values of F_a and F_r, and value of C₀ of selected bearing. Refer table 28.2 for deep groove ball bearings.
6. Calculate equivalent dynamic load of the bearing,

7. Decide expected life of the bearing in millions of revolutions (L).

8. Calculate the required dynamic load carrying capacity for expected life, using load life relationship.

9.Dynamic load carrying capacity of the selected bearing should be greater than the required value calculated above, i.e.

If , choose higher series of the bearings from the catalogue and repeat the procedure from Step 3.

 

References

1. Design of Machine Elements by VB Bhandari
2. Analysis and Design of Machine Elements by V.K. Jadon
3. Machine Design by R.S. Khurmi
4. Design of Machine Elements by C.S. Sharma & K. Purohit
5. 5.      SKF General Catalogue