Site pages
Current course
Participants
General
MODULE 1. FLUIDS MECHANICS
MODULE 2. PROPERTIES OF FLUIDS
MODULE 3. PRESSURE AND ITS MEASUREMENT
MODULE 4. PASCAL’S LAW
MODULE 5. PRESSURE FORCES ON PLANE AND CURVED SUR...
MODULE 6.
MODULE 7. BUOYANCY, METACENTRE AND METACENTRIC HEI...
MODULE 8. KINEMATICS OF FLUID FLOW
MODULE 9: CIRCULATION AND VORTICITY
MODULE 10.
MODULE 11.
MODULE 12, 13. FLUID DYNAMICS
MODULE 14.
MODULE 15. LAMINAR AND TURBULENT FLOW IN PIPES
MODULE 16. GENERAL EQUATION FOR HEAD LOSS-DARCY EQ...
MODULE 17.
MODULE 18. MAJOR AND MINOR HYDRAULIC LOSSES THROUG...
MODULE 19.
MODULE 20.
MODULE 21. DIMENSIONAL ANALYSIS AND SIMILITUDE
MODULE 22. INTRODUCTION TO FLUID MACHINERY
LESSON 26. EMPRICAL EQUATIONS FOR FRICTION HEAD LOSS
Hazen-Williams equation:
It was developed for water flow in larger pipes (D≥5 cm, approximately 2 in.) within a moderate range of water velocity (V≤3 m/s, approximately 10 ft/s). Hazen-Williams equation, originally developed for the British measurement system, has been written in the form
![]()
S= slope of the energy grade line, or the head loss per unit length of the pipe (S=hf/L)
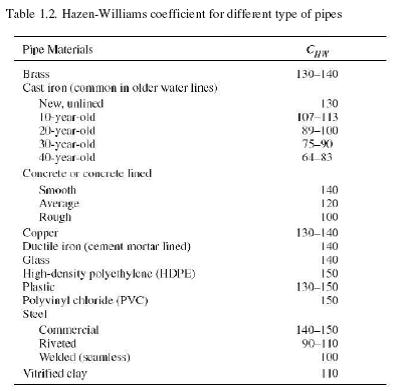
Rh = the hydraulic radius, defined as the water cross sectional area (A) divided by wetted perimeter (P). For a circular pipe, with A=πD2/4 and P=πD, the hydraulic radius is
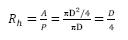
CHW= Hazen-Williams coefficient. The values of CHW for commonly used water-carrying conduits are given in Table 1.2.
The Hazen-Williams equation in SI units is written in the form of
![]()
Velocity in m/s and Rh is in meters
Manning’s Equation
Manning equation has been used extensively open channel designs. It is also quite commonly used for pipe flows. The Manning equation may be expressed in the following form:
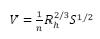
n= Manning’s coefficient of roughness. Typical values of n for water flow in common pipe materials is given in Table 1.3
In British units, the Manning equation is written as
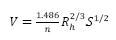
Where V is units of ft/s.