Fluid Mechanics 3(2+1)

LESSON 30. DIMENSIONAL ANALYSIS

The area where dimensional analysis is used are:

• design experiments

• Informs which measurements are important

• Allows most to be obtained from experiment:

e.g. What runs to do. How to interpret.

• It depends on the correct identification of variables

• Relates these variables together

• Doesn’t give the complete answer

• Experiments necessary to complete solution

• Uses principle of dimensional homogeneity

• Give qualitative results which only become quantitative from experimental analysis.

Dimensions and units

• Any physical situation can be described by familiar properties.

e.g. length, velocity, area, volume, acceleration etc.

• These are all known as dimensions.

• Dimensions are of no use without a magnitude.

i.e. a standardised unit e.g metre, kilometre, Kilogram, a yard etc.

• Dimensions can be measured.

• Units used to quantify these dimensions.

•  In dimensional analysis we are concerned with the nature of the dimension i.e. its quality not its quantity.

The following common abbreviations are used:

Length  [L]

Area [L²]

Mass  [M]

Time  [θ]

Force  [F]

Temperature [T]

• Here we will use L, M, T and F.

• We can represent all the physical properties we are interested in with three:

L, T and one of M or F

• As either mass (M) of force (F) can be used to represent the other, i.e.

F = MLT^-2

M = FT2L^-1

• We will always use LTM:

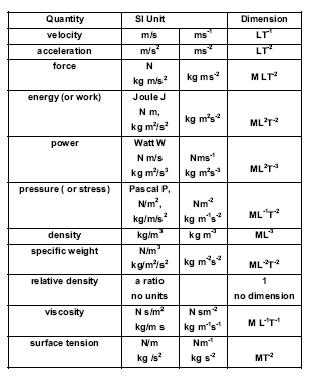
• This table lists dimensions of some common physical quantities:

The buckingham pi theorem

The buckingham pi theorem puts the ‘method of dimensions’ first proposed by lord rayleigh in his book “the theory of sound” (1877) on a solid theoretical basis, and is based on ideas of matrix algebra and concept of the ‘rank’ of non-square matrices which you may see in math classes. although it is credited to E. Buckingham (1914), in fact, white points out that the theorem has also appeared earlier in independent publications by a. vaschy (1892) and d. riabouchinsky (1911).

The Theorem

Let q1,q2,q3,……qn be n dimensional variables that are physically relevant n a given problem and that are interrelated by an unknown) dimensionally homogeneous set of equations. These can be expressed via a functional relationship of the form:

F(q1,q2,...qn)=0

q1=f(q2,...qn)

If k is the number of fundamental dimensions required to describe the n variables, then there will be k primary variables and the remaining j=(n-k) variables can be expressed as (n-k) dimensionless and independent quantities or pi groups Π₁, Π₂,…. Π_n-k. The functional relationship can thus be reduced to the much more compact form:

ɸ(Π₁, Π₂,…. Π_n-k)=0                  or equivalently                       Π₁= ɸ (Π₂,…. Π_n-k).

 

Applications of Buckingham pi Theorem

i) Clearly define the problem and think about which variables are important. Identify which is the main variable of interest i.e. q₁=f(q₂…q_n). It is important to think physically about the problem. Are there any constraints; i.e. ‘can I vary all of these variables independently’;

e.g. weight of an object F_w=ρgl³ (only two of these are independent, unless g is also variable)

ii) Express each of n variable in terms of its fundamental dimensions, {MLTθ} or {FLTθ}It is often useful to use one system to do problem, and then check that groups you obtain are dimensionless by converting to other system.

iii) Determine the number of Pi groups j=n-k, where k is the number of reference dimensions and select k primary or repeating variables. Typically pick variables which characterize the fluid properties, flow geometry, flow rate…

iv) Form j dimensionless Π groups and check that they are all indeed dimensionless.

v) Express result in form Π₁= ɸ₁ (Π₂,…. Π_n-k) where Π₁ contains the quantity of interest and interpret your result physically!

vi) Make sure that your groups are indeed independent; i.e. can I vary one and keep others constant.

vii) Compare with experimental data!