Electrical Circuits 3(2+1)

LESSON 27. Parallel Resonance

In fig. 26.1, the total admittance

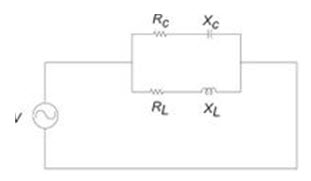
Fig. 27.1

\[Y={1 \over {{R_L} + j\omega L}} + {1 \over {{R_C} - \left( {j/\omega C} \right)}}\]

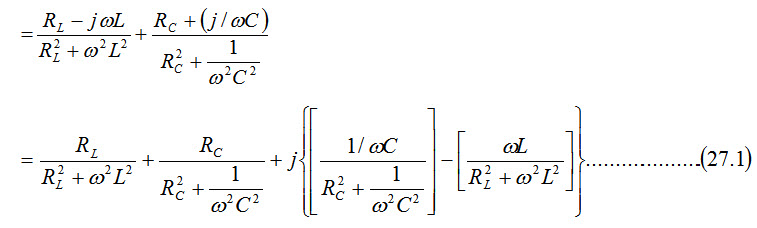
 At resonance the susceptance part becomes zero

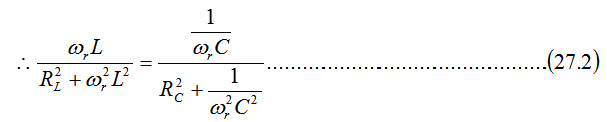
\[{\omega _r}L\left[ {R_C^2 + {1 \over {\omega _r^2{C^2}}}} \right]={1 \over {{\omega _r}C}}\left[ {R_L^2 + \omega _r^2{L^2}} \right]\]

\[\omega _r^2\left[ {R_C^2 + {1 \over {\omega _r^2{C^2}}}} \right] = {1 \over {LC}}\left[ {R_L^2 + \omega _r^2{L^2}} \right]\]

\[\omega _r^2R_C^2 - {{\omega _r^2} \over C}={1 \over {LC}}R_L^2 - {1 \over {{C^2}}}\]

\[\omega _r^2\left[ {R_C^2 - {L \over C}} \right]={1 \over {LC}}\left[ {R_L^2 - {L \over C}} \right]\]

\[{\omega _r}={1 \over {\sqrt {LC} }}\sqrt {{{R_L^2 - \left( {L/C} \right)} \over {R_C^2 - \left( {L/C} \right)}}} ...................................................\left( {27.3} \right)\]

The condition for resonant frequency is given by EQ.8.16. As a special case, if R_L = R_C, then Eq.27.3 becomes

\[{\omega _r}={1 \over {\sqrt {LC} }}\]

Therefore        \[{f_r}={1 \over {2\pi \sqrt {LC} }}\]

27.2. Resonant Frequency for a Tank Circuit

The parallel resonant circuit is generally called a tank circuit because of the fact that the circuit stores energy in the magnetic field of the coil and in the electric field of the capacitor.  The stored energy is transferred back and forth between the capacitor and coil and vice-versa.  The tank circuit is shown in Fig.27.2.  The circuit is said to be in resonant condition when the susceptance part of admittance is zero.

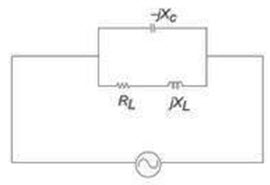
Fig.27.2

The total admittance is

\[Y={1 \over {{R_L} + j{X_L}}} + {1 \over { - j{X_C}}}...................................................\left( {27.4} \right)\]

Simplifying Eq. 27.4, we have

\[Y={{{R_L} - j{X_L}} \over {R_L^2 + X_L^2}} + {j \over {{X_C}}}\]

\[={{{R_L}} \over {R_L^2 + X_L^2}} + j\left[ {{1 \over {{X_C}}} - {{{X_L}} \over {R_L^2 + X_L^2}}} \right]\]

To satisfy the condition for resonance, the susceptance part is zero.

\[{1 \over {{X_C}}}={{{X_L}} \over {R_L^2 + X_L^2}}...................................................\left( {27.5} \right)\]

\[\omega C={{\omega L} \over {R_L^2 + {\omega ^2}{L^2}}}...................................................\left( {27.6} \right)\]

From Eq.27.6, we get

\[R_L^2 + {\omega ^2}{L^2}={L \over C}\]

\[{\omega ^2}{L^2}={L \over C} - R_L^2\]

\[{\omega ^2}={1 \over {LC}} - {{R_L^2} \over {{L^2}}}\]

\[\,\omega=\sqrt {{1 \over {LC}} - {{R_L^2} \over {{L^2}}}} ...................................................\left( {27.7} \right)\]

The resonant frequency for the tank circuit is

\[{f_r}={1 \over {2\pi }}\sqrt {{1 \over {LC}} - {{R_L^2} \over {{L^2}}}} ...................................................\left( {27.8} \right)\]

27.3. Variation of Impedance with Frequency

The impedance of a parallel resonant circuit is maximum at the resonant frequency and decreases at lower and higher frequencies as shown in Fig. 27.3.

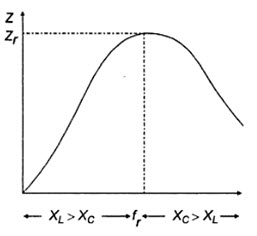
Fig.27.3

At very low frequencies, X_L is very small and X_C is very large, so the total impedance is essentially inductive.  As the frequency increases, the impedance also increases, and the inductive reactance dominates until the resonant frequency is reached.  At this point X_L=X_C and the impedance is at its maximum.  As the frequency goes above resonance, capacitive reactance dominates and the impedance decreases.

27.4. Q Factor of Parallel Resonance

Consider the parallel RLC circuit shown in Fig. 27.4.

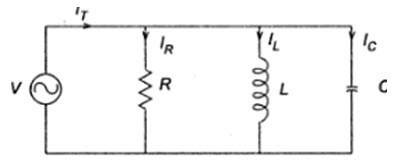
Fig.27.4

In the circuit shown, the condition for resonance occurs when the susceptance part is zero.

Admittance Y = G + jB \[...................................................\left( {27.9} \right)\]

       \[={1 \over R} + j\omega C + {1 \over {j\omega L}}\]

\[={1 \over R} + j\left( {\omega C + {1 \over {\omega L}}} \right)...................................................\left( {27.10} \right)\]

The frequency at which resonance occurs is

\[{\omega _r}C - {1 \over {{\omega _r}L}}=0...................................................\left( {27.11} \right)\]

The voltage and current variation with frequency is shown in Fig. 27.5. At resonant frequency, the current is minimum.

The bandwidth, BW=f₂-f₁

For parallel circuit, to obtain the lower power half frequency,

\[{\omega _1}C - {1 \over {{\omega _1}L}}={1 \over R}...................................................\left( {27.12} \right)\]

From Eq.27.12, we get

\[\omega _1^2 + {{{\omega _1}} \over {RC}} - {1 \over {LC}}=0...................................................\left( {27.13} \right)\]

If we simplify Eq.27.13, we get

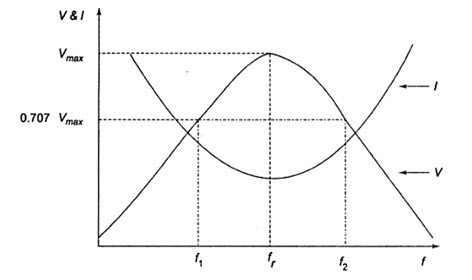
Fig.27.5

\[{\omega _1}={{ - 1} \over {2RC}} + \sqrt {{{\left( {{1 \over {2RC}}} \right)}^2} + {1 \over {LC}}} ...................................................\left( {27.14} \right)\]

Similarly, to obtain the upper half power frequency

\[{\omega _2}C - {1 \over {{\omega _2}L}}={1 \over R}...................................................\left( {27.15} \right)\]

From Eq.27.15, we have

\[{\omega _2}={1 \over {2RC}} + \sqrt {{{\left( {{1 \over {2RC}}} \right)}^2} + {1 \over {LC}}} ...................................................\left( {27.16} \right)\]

Bandwidth  \[BW={\omega _2} - {\omega _1}={1 \over {RC}}\]

The quality factor is defined as           \[{Q_r}={{{\omega _r}} \over {{\omega _2}{\omega _1}}}\]

\[{Q_r}={{{\omega _r}} \over {1/RC}}={\omega _r}RC\]

In other words,

\[Q=2\pi\times {{\max imum\,\,energy\,\,stored} \over {Energy\,\,dissipated\,\,/\,cycle}}\]

In the case of an inductor,

The maximum energy stored \[={1 \over 2}L{I^2}\]

Energy dissipated per cycle   \[={\left( {{I \over {\sqrt 2 }}} \right)^2} \times R \times T\]

The quality factor       

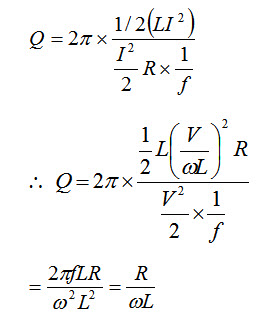
For a capacitor, maximum energy stored = ½ (CV²)

Energy dissipate per cycle \[=P \times T={{{V^2}} \over {2 \times R}} \times {1 \over f}\]

The quality factor 

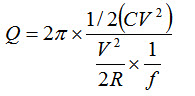
\[=2\pi fCR=\omega CR\]