Soil Mechanics

LESSON 27. Rankine’s Theory of Earth Pressure and Numerical Exercise

where c is the cohesion of the soil, \[\gamma\] is the effective unit weight of the soil and K_A is the coefficient of active earth pressure and can be expressed as:

\[{K_A}={{1 - \sin \phi } \over {1 + \sin \phi }}\]                       (27.2)

During the calculation of total active force (P_A) in case of c-ø backfill, negative zone is neglected and only the active earth pressure due to the positive zone is considered. Thus,

\[{P_A}={1\over 2}{K_A}\gamma\,{\left({H-{Z_0}}\right)^2}={1\over 2}{K_A}\gamma\,{H^2}-2cH\sqrt{{K_A}}+{{2{c^2}}\over\gamma}\]         (27.3)

P_A acts at the height of (H-Z₀)/3 from the base of the wall.

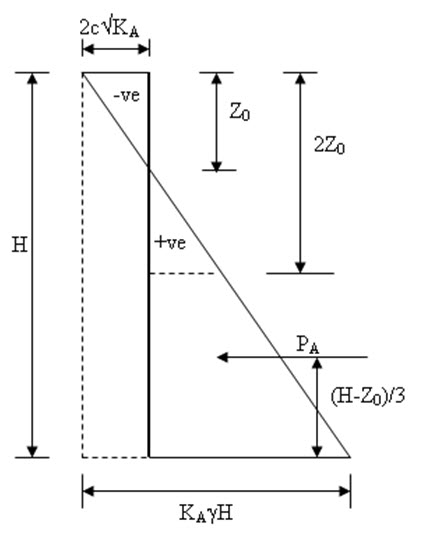
Fig. 27.1. Active earth pressure for c-ø backfill.

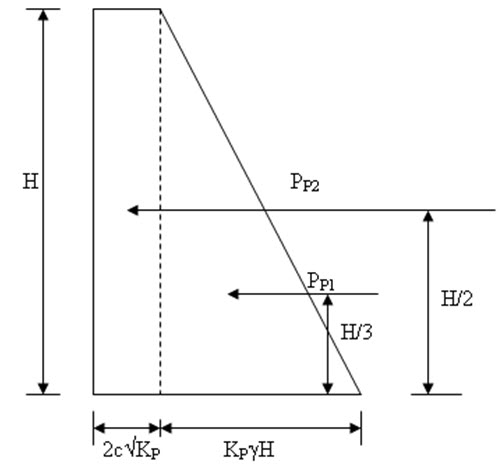
Fig. 27.2. Passive earth pressure for c-ø backfill.

Figure 27.2 shows the passive earth pressure distribution for c-ø backfill. The passive force P_P1 and P_P2 can be determined as:

The passive force P_P1 and P_P2 can be determined as:

\[{P_{P1}}={1 \over 2}\gamma\,{K_P}{H^2}\] acts at a height of H/3 from the base                             (27.4)

\[{P_{P2}}=2cH\sqrt{{K_P}}\] acts at a height of H/2 from the base                    (27.5)

          Thus the total force P_P can be determined as:

\[{P_P}={1\over 2}\gamma {K_P}{H^2} + 2cH\sqrt{{K_P}}\]                                                                      (27.6)

where  \[{K_P}={{1 + \sin \phi}\over{1-\sin\phi}}\]

Problem

Determine the active earth pressure distribution on the retaining as shown in Figure 27.3. Also determine the total active force and point of application  of the force.

Solution:

K_A1 for layer I = \[{K_A}={{1-\sin\phi}\over{1+\sin\phi}}\] = \[{{1-\sin 28^\circ}\over{1+\sin 28^\circ}}\] = 0.36

K_A2 for layer II = \[{K_A}={{1-\sin\phi}\over{1+\sin\phi}}\] = \[{{1-\sin 32^\circ}\over{1+\sin 32^\circ}}\ = 0.31

Active Pressure distribution for layer I

At Z = 0m, P_A = 0 kN/m²

At Z = 1.5m, P_A = 0.36 x 18 x 1.5 = 9.72 kN/m²

At Z = 6 m, P_A (due to soil) = 9.72 + 0.36 x (20 – 10) x 4.5 = 9.72 + 16.2 =25.92 kN/m² (take unit weight of the water is 10 kN/m³).

At Z = 6 m, P_A (due to water) = 4.5 x 10 = 45 kN/m²

Active Pressure distribution for layer II.

At Z = 6 m, P_A (due to surcharge of the layer I) = 0.31 (1.5 x 18 + 4.5 x 10) = 22.32 kN/m²

At Z = 6 m, P_A (due to the water Pressure above this level) = 45 kN/m²

At Z = 11 m, P_A (due to soil) = 67.32 + 0.31 x (20 – 10) x 5 = 67.32 + 15.5 =82.82 kN/m²

At Z = 6 m, P_A (due to water) = 5 x 10 = 50 kN/m²

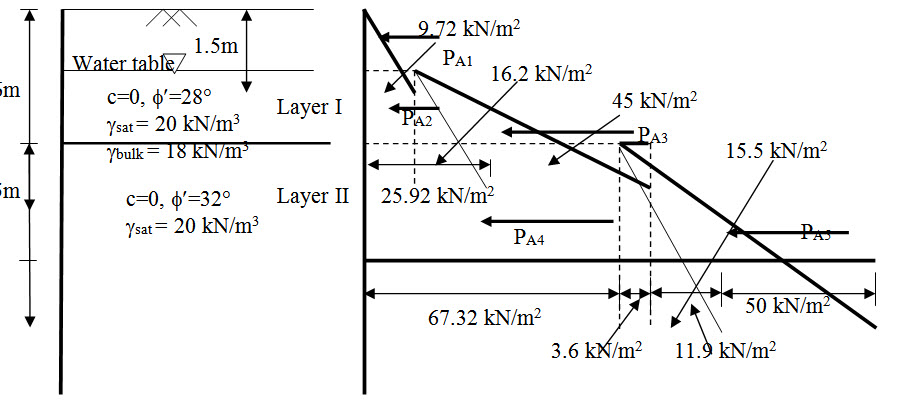
Fig. 27.3. Active earth Pressure distribution of problem 1.

The active force of the various levels is calculated as:

P_A1 = 0.5 x 9.72 x 1.5 = 7.29 kN/m acts at a height of 10 (=1.5/3+4.5+5) m from the base

P_A2 = 9.72 x 4.5 = 43.74 kN/m acts at a height of 7.25 (=4.5/2+5) m from the base

P_A3 = 0.5 x (16.2 + 45) x 4.5 = 137.7 kN/m acts at a height of 6.5 (=4.5/3+5) m from the base

P_A4 = 67.32 x 5 = 336.6 kN/m acts at a height of 2.5 (=5/2) m from the base

P_A5 = 0.5 x (15.5 + 50) x 5 = 163.75 kN/m acts at a height of 1.67 (=5/3) m from the base

P_A = P_A1+ P_A2 + P_A3+ P_A4+ P_A5 = 7.29 + 43.74 + 137.7 + 336.6 + 163.75 = 689.08 kN/m

Point of aPPlication of the resultant force P_A is

      

 

References

Ranjan, G. and Rao, A.S.R. (2000). Basic and Applied Soil Mechanics. New Age International Publisher, New Delhi, India.

 

Suggested Readings

Ranjan, G. and Rao, A.S.R. (2000) Basic and Applied Soil Mechanics. New Age International Publisher, New Delhi, India.

Arora, K.R. (2003) Soil Mechanics and Foundation Engineering. Standard Publishers Distributors, New Delhi, India.

Murthy V.N.S (1996) A Text Book of Soil Mechanics and Foundation Engineering, UBS Publishers’ Distributors Ltd. New Delhi, India.