Site pages
Current course
Participants
General
MODULE 1. BASIC CONCEPTS
MODULE 2. SYSTEM OF FORCES
MODULE 3.
MODULE 4. FRICTION AND FRICTIONAL FORCES
MODULE 5.
MODULE 6.
MODULE 7.
MODULE 8.
19 April - 25 April
26 April - 2 May
LESSON 11. RADIUS OF GYRATION OF AN AREA ABOUT AN AXIS
11.1 RADIUS OF GYRATION OF AN AREA ABOUT AN AXIS
Consider an area A whose moment of inertia about x and y axes is Ix and Iy. Suppose the area concentrated into a thin strip parallel of to x-axis as shown in Fig.11.1. such that its moment of inertia about x-axis is same as Ix. Then the distance at which this strip is to be placed from x-axis is called radius of gyration of the area about x-axis, denoted by Rx.
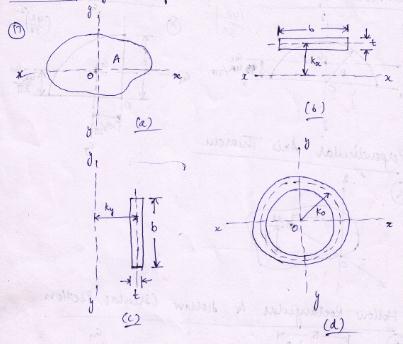
Fig.11.1 Radius of gyration
According to the parallel axis theorem, the moment of inertia of the strip about x-axis is
Ixx = Icent + ARx2 (11.19)
But Icent = \[{{b{t^3}} \over {12}}\] is negligible as t is small, t3 is negligibly smaller.
Therefore,
Ixx = ARx2
Or Rx = \[\sqrt {{{{I_{xx}}} \over A}}\] (11.20)
It is expressed in mm or cm or m.
Hence, the radius of gyration of an area about an axis is defined as the distance from the axis where thin strip (having an area equal to the given area) is to be placed so that the moment of inertia of the strip about the axis is equal to the moment of inertia of the given area about that axis.
Similar to Eq. (11.20), the radius of gyration of the area about y-axis [Figure 11.13(c)] and pole or polar radius of gyration [Figure 11.13(d)] are obtained as given in Eqs. (11.21) and (11.22).
Ry = \[\sqrt {{{{I_{yy}}} \over A}} \] (11.21)
R0 = \[\sqrt {{J \over A}}\] (11.22)
Where J = polar moment of inertia using perpendicular axis theorem
J = Ixx + Iyy
AR02 = ARx2 + ARy2
Hence
R02 = Rx2 + Ry2 (11.23)
Example 1: Find the moment of inertia of the rectangular section as shown in Figure------- about the faces PQ and RS.
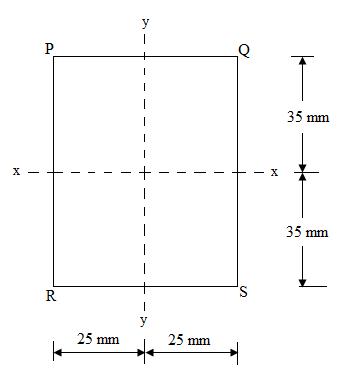
Fig.11.2
Sol: From the Figure, width b = 50 mm and depth d = 70 mm
The moment of inertia of the entire rectangular area about PQ is given by
IPQ = Ixx + Ah2
= \[{{b{d^3}} \over {12}} + \left( {b \times d} \right){h^2}\]
= \[{{50 \times {{70}^3}} \over {12}} + \left( {50 \times 70} \right) \times {\left( {35} \right)^2}\]
=1429166.67 + 4287500
= 5716666.67 mm4
IBC = Iyy + Ah2
= \[{{b{d^3}} \over {12}} + \left( {b \times d} \right){h^2}\]
= \[{{70 \times {{50}^3}} \over {12}} + \left( {70 \times 50} \right) \times {\left( {25} \right)^2}\]
= 729166.67+2187500
= 2916666.67 mm4
Example 2: Find the moment of inertia of a hollow rectangular section about two mutually perpendicular axes in its plane and passing through its centroid . The dimensions of the outer rectangle are 80 mm×100 mm and that of the inner rectangle is 60 mm×80 mm.
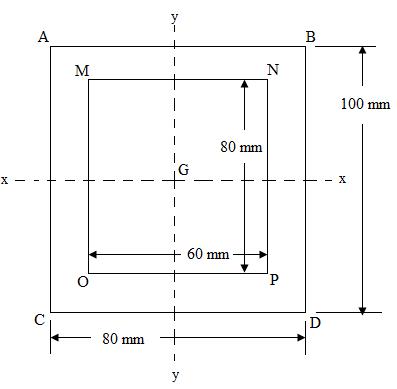
Fig.11.3
Sol: Outer rectangle: B = 80 mm and D = 100 mm
Inner rectangle: b = 60 mm and d = 80 mm
The formula is IGx = \[{1 \over {12}}\left( {B{D^3} - b{d^3}} \right)\]
= \[{1 \over {12}}\left( {80 \times {{100}^3} - 60 \times {{80}^3}} \right)\]
= 4106666.67 mm4
IGy = \[{1 \over {12}}\left( {D{B^3} - d{b^3}} \right)\]
= \[{1 \over {12}}\left( {100 \times {{80}^3} - 80 \times {{60}^3}} \right)\]
= 2826666.67 mm4
Example 3: Determine the moment of inertia and radius of gyration about the horizontal axis passing through the centroid of the section as shown in Figure.
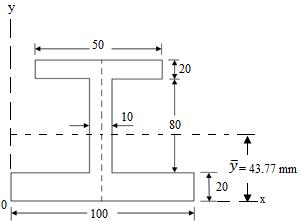
Fig.11.4
Sol: The given I-section is symmetrical about the y-y axis, therefore,
\[\bar x = {{100} \over 2}\] = 50 mm
To find , we have to divide the whole Figure into standard areas.