Engineering Mechanics

LESSON 29.

29.1.1 Mohr’s circle construction for ‘like stresses’

Steps of construction:

Using some suitable scales, measure OL and OM equal to σ_x and σ_y respectively on the axis OX.

1. Bisect LM at N.

2. With N as centre and NL or NM radius, draw a circle.

3. At the centre N draw a line NP at an angle 2Ө, in the same direction as the normal to the plane makes with the direction of σ_x. In Fig.1 which represents the stress system, the normal to the plane makes an angle Ө with the direction of σ_x in the anticlockwise direction. The line NP therefore, is drawn in the anticlockwise direction.

4. From P, draw a perpendicular PQ on the axis OX. PQ will represent τ and OQ σ_n.

Now, from stress diagram

NP = NL = \[{{{\sigma _{x - {\sigma _y}}}} \over 2}\]

PQ = NP sin2Ө = \[{{{\sigma _{x - {\sigma _y}}}} \over 2}\]  sin2Ө = τ ----------------------------------------(8.1)

Similarly, OQ = ON + NQ = \[{{{\sigma _{x + {\sigma _y}}}} \over 2}\]  + \[{{{\sigma _{x - {\sigma _y}}}} \over 2}\]  cos2Ө = σ_n--------------(8.2)

Also, from stress circle, τ is maximum when

2Ө = 90°, or Ө = 45°

And, τ_max = \[{{{\sigma _{x - {\sigma _y}}}} \over 2}\]  ----------------------------------------------------(8.3)

Sign conventions used:

(i) In order to mark τ in stress system, we will take the clockwise shear as positive and anticlockwise shear as negative.

(ii) Positive value of τ will be above the axis and negative values below the axis.

(iii) If Ө is in the anticlockwise direction, the radius vector will be above the axis and Ө will be reckoned positive. If Ө is in the clockwise direction, it will be negative and the radius vector will be below the axis.

(iv) Tensile stress will be reckoned positive and will be plotted to the right of the origin O. Compressive stress will be reckoned negative and will be plotted to the left of the origin O.

29.1.2 Mohr’s circle construction for ‘Unlike stresses’

In case σ_x and σ_y are not like, the same procedure will be followed except that σ_x and σ_y will be measured to the opposite sides of the origin. The construction is given in Fig.2.It may be noted that the direction of σ_n will depend upon its position with respect to the point O. If it is to the right of O, the direction of σ_n will be the same as that of σ_x.

 

29.1.3 Mohr’s circle construction for two perpendicular direct stresses with state of simple shear

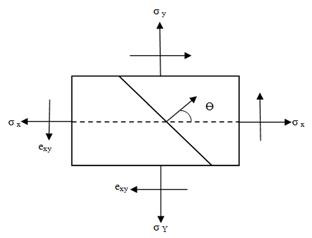
Refer to Fig.3. Following steps of construction are followed if the material is subjected to direct stresses σ_x and σ_y along with a state of simple shear.

(a)

(b)

Fig.29.3

1. Using some suitable scale, measure OL = σ_x and OM = σ_y along the axis OX.

2. At L draw LT perpendicular to OX and equal to τ_xy. LT has been drawn downward (as per sign conventions adopted) because τ_xy is acting up with respect to the plane across which σ_x is acting, tending to rotate it in the anticlockwise direction and is negative.

3. Similarly, make MS perpendicular to OX and equal to τ_xy, but above OX.

4. Join ST to cut the axis in N.

5. With N as centre and NS or NT as radius, draw a circle.

6. At N make NP at angle 2Ө with NT in the anticlockwise direction.

7. Draw PQ perpendicular to the axis. PQ will give τ while OQ will give σ_n and OP will give σ_r.

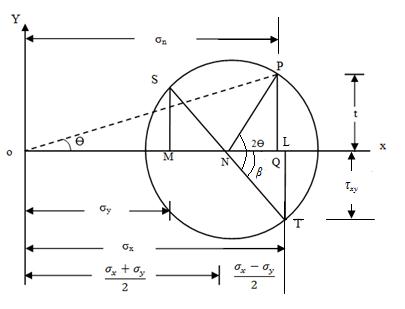
Proof: Let the radius of the stress circle be R.

Then,        R = \[\sqrt {N{L^2} + L{T^2}}\]  =  \[\sqrt {{{\left[ {{{{\sigma _x} - {\sigma _y}} \over 2}} \right]}^2} + {\tau _{xy}}^2}\]

Also,    R cos β = NL =  \[{{{\sigma _{x - {\sigma _y}}}} \over 2}\]

          R sin β = LT = τ_xy

Now,     OQ = ON + NQ = ON + R cos (2Ө – β)

                     = ON + R cos 2Ө cos β + R sin 2Ө sin β

                     = \[{{{\sigma _{x + {\sigma _y}}}} \over 2}\] + \[{{{\sigma _{x - {\sigma _y}}}} \over 2}\]  cos2Ө + τ_xy sin 2Ө   (as per eqn-------)

                     = σ_n

Similarly,

PQ = R sin (2Ө – β) = R sin2Ө cos β – R cos2Ө sin β

       = \[{{{\sigma _{x - {\sigma _y}}}} \over 2}\]  sin2Ө – τ_xy cos 2Ө                                  (as per eqn-------)

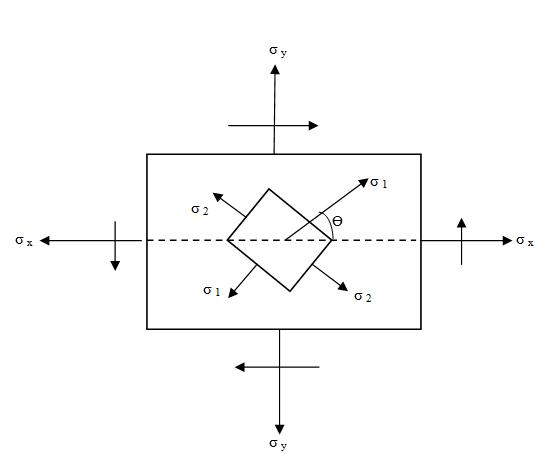
 29.1.4 Mohr’s circle construction for principal stresses

Refer to Fig.4.The following are the steps of construction:

(a)

 

(b)

Fig. 29.4

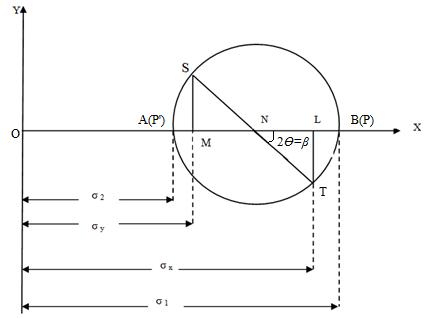
1. Mark OL and OM proportional to σ_x and σ_y.

2. At L and M, erect perpendiculars LT = MS proportional to  in appropriate directions.

3. Join ST, intersecting the axis in N.

Since τ = o, NV represents the major principal plane, P coinciding with B. Similarly NPꞌ represents minor principal plane, Pꞌ coinciding with A.

OV = ON + NV = \[{{{\sigma _{x + {\sigma _y}}}} \over 2}\]  + R,        where R is the radius of the circle.

       = \[{{{\sigma _{x - {\sigma _y}}}} \over 2}\] + \[\sqrt {{{\left[ {{{{\sigma _x} - {\sigma _y}} \over 2}} \right]}^2} + {\tau _{xy}}^2}\] = σ₁

Similarly,      OU = ON – NU

                            = \[{{{\sigma _{x + {\sigma _y}}}} \over 2}\] - \[\sqrt {{{\left[ {{{{\sigma _x} - {\sigma _y}} \over 2}} \right]}^2} + {\tau _{xy}}^2}\]  = σ₂

tan β =\[\frac{{LT}}{{LN}}\] = \[\frac{{\tau xy}}{{\frac{{{\sigma _x} - {\sigma _y}}}{2}}}\]

       = \[{{2\tau xy} \over {{\sigma _x} - {\sigma _y}}}\] = tan 2Ө             (where β = 2Ө)