LESSON 32.

32.1 PRINCIPAL PLANES AND PRINCIPAL STRESSES

  • A body may be subjected to stresses in one plane or in different planes.

  • There are always three mutually perpendicular planes along which the stresses at a certain point (in a body) can be resolved completely into stresses normal to these planes.   

  • These planes which pass through the point in such a manner that the resultant stress across them is totally a normal stress are known as “Principal planes” and normal stresses across these planes are termed as “principal stresses”.

  • The plane carrying the maximum normal stress is called the major principal planes and the corresponding stress the major principal stress. The plane carrying the minimum normal stress is known as major principal plane and the corresponding stress as major principal stress.

Example: The principal stresses in the wall of container (Fig.32.1) are 40 kN/m2 and 80 kN/m2. Determine the normal, shear and resultant stresses in  magnitude and direction in a plane, the normal of which makes an angle of 30° with the direction of maximum principal stresses.

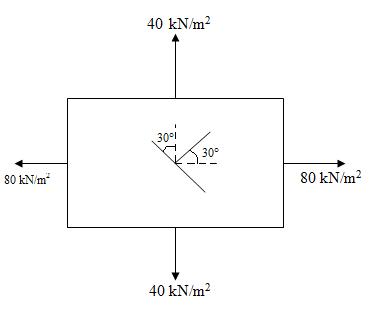
Module 8 Lesson 32 Fig.1.1

Solution: Given: σx = 80 kN/m2 (tensile);

σy = 40 kN/m2 (tensile);

Ө = 30°

(i) Normal stress, σn :

σn = \[{{{\sigma _{x + {\sigma _y}}}} \over 2}\] + \[{{{\sigma _{x - {\sigma _y}}}} \over 2}\]  cos2Ө...........................(2.1)

     = \[{{80 + 40} \over {2}} + {{80 - 40} \over 2}\] cos60°

     = 60 + 10 = 70 kN/m2

(ii) Shear stress, τ :

τ = \[{{{\sigma _{x - {\sigma _y}}}} \over 2}\] sin2Ө =  \[{{80 + 40} \over {2}}\]  sin60°....................(2.2)

   = 17.32 kN/m2

(iii) Resultant stress, σr , ϕ:

σr = \[\sqrt {{\sigma _n}^2 + {\tau ^2}}\] = \[\sqrt {{{70}^2} + {{17.32}^2}}\]

i.e, σr = 72.11 kN/m2

If ϕ is the angle that the resultant makes with the normal to the plane, then

tanϕ = \[{\tau\over {{\sigma _n}}}\] = \[{{17.32} \over {70}}\]  = 0.2474

ϕ = 13°54ꞌ

Last modified: Wednesday, 11 September 2013, 8:55 AM