Fluid Mechanics 3(2+1)

Lesson-13: FLOW VISUALIZATION

13.1.2 Non-uniform flow

If at a given instant, the velocity is not the same at every point the flow. (In practice, by this definition, every fluid that flows near a solid boundary will be non-uniform - as the fluid at the boundary must take the speed of the boundary, usually zero. However if the size and shape of the of the cross-section of the stream of fluid is constant the flow is considered uniform.)

13.1.3 Steady flow

A steady flow is one in which the conditions (velocity, pressure and cross-section) may differ from point to point but DO NOT change with time.

13.1.4 Unsteady flow

If at any point in the fluid, the conditions change with time, the flow is described as unsteady. (In practice there is always slight variations in velocity and pressure, but if the average values are constant, the flow is considered steady.)

13.1.5 Steady uniform flow

Conditions: do not change with position in the stream or with time.

Example: the flow of water in a pipe of constant diameter at constant velocity.

13.1.6 Steady non-uniform flow

Conditions: change from point to point in the stream but do not change with time.

Example: flow in a tapering pipe with constant velocity at the inlet-velocity will change as you move along the length of the pipe toward the exit.

13.1.7 Unsteady uniform flow

At a given instant in time the conditions at every point are the same, but will change with time.

Example: a pipe of constant diameter connected to a pump pumping at a constant rate which is then switched off.

13.1.8 Unsteady non-uniform flow

Every condition of the flow may change from point to point and with time at every point.

Example: waves in a channel.

13.1.9 Laminar flow

All the particles proceed along smooth parallel paths and all particles on any path will follow it without deviation.

Hence all particles have a velocity only in the direction of flow.

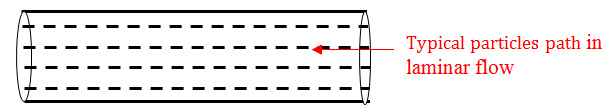
13.1.10 Turbulent Flow

The particles move in an irregular manner through the flow field.

Each particle has superimposed on its mean velocity fluctuating velocity components both transverse to and in the direction of the net flow.

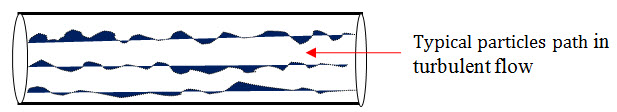
13.1.11 Transition Flow

• Exists between laminar and turbulent flow.

• In this region, the flow is very unpredictable and often changeable back and forth between laminar and turbulent states.

• Modern experimentation has demonstrated that this type of flow may comprise short ‘burst’ of turbulence embedded in a laminar flow.

• Rotational flow is the type of flow in which the fluid particles while flowing along stream-lines also rotate about their own axis.

•  Irrotational flow is the type of flow in which the fluid particles while flowing along stream-lines do not rotate about their own axis.

13.1.12 Compressible or Incompressible flow

• All fluids are compressible - even water - their density will change as pressure changes.

• Under steady conditions, and provided that the changes in pressure are small, it is usually possible to simplify analysis of the flow by assuming it is incompressible and has constant density.

• As you will appreciate, liquids are quite difficult to compress - so under most steady conditions they are treated as incompressible.

13.1.13 One, Two or Three-dimensional Flow

• In general, all fluids flow three-dimensionally, with pressures and velocities and other flow properties varying in all directions.

• In many cases the greatest changes only occur in two directions or even only in one.

• In these cases changes in the other direction can be effectively ignored making analysis much more simple.

• Flow is one dimensional if the flow parameters (such as velocity, pressure, depth etc.) at a given instant in time only vary in the direction of flow and not across the cross-section. The flow may be unsteady, in this case the parameter vary in time but still not across the cross-section.

• Flow is two-dimensional if it can be assumed that the flow parameters vary in the direction of flow and in one direction at right angles to this direction.

13.1.14 ROTATIONAL AND IRROTATIONAL FLOWS

• Rotational flow is the type of flow in which the fluid particles while flowing along stream-lines also rotate about their own axis.

•  Irrotational flow is the type of flow in which the fluid particles while flowing along stream-lines do not rotate about their own axis.