Watershed Hydrology 3(2+1)

Lesson 7 Estimation of Missing Rainfall Data

 

1. Simple Arithmetic Method

2. Normal Ratio Method

3. Modified normal ratio method

4. Inverse distance method

5. Linear programming method

For m stations, 1, 2, 3, …,m, the annual precipitation values are P1, P2, P3, …, Pm, respectively. At station x (not included in the above m stations), the missing annual precipitation (Px) should be found out.  The normal annual precipitation N1, N2, N3, …,Ni at each of the above (m+1) stations including the station x is known.

 

7.1.1 Normal Precipitation - It is the average value of precipitation at a particular date, month or year over a specified 30 year period.  Thus, the term normal annual precipitation at station A means the average annual precipitation at A based on a specified 30 year of record.

 

7.1.2 Simple Arithmetic Average - The missing precipitation Px can be determined using simple arithmetic average, if the normal annual precipitation at various stations are within 10% of the normal precipitation at station, x, as follows:

                                                                   (7.1)

7.1.3 Normal Ratio Method - If the normal precipitations vary considerably then Px is estimated by weighting the precipitation at various stations by the ratios of normal annual precipitation.  The normal ration method gives Px as:

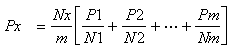                                                          (7.2)

This method is based selecting m (m is usually 3) stations that are near and approximately evenly spaced around the station with the missing record.

 

Example 7.1 The normal annual rainfall at stations A, B, C and D in a basin are 80.97, 67.59, 76.28, and 92.01 cm, respectively.  In the year 1975, the station D was inoperative and the stations A, B, and C recorded annual rainfall of 91.11, 72.23, and 79.89 cm, respectively.  Estimate the rainfall at station D in that year.

 

Solution:  As the normal rainfall values vary by more than 10%, the ration method is adopted.

 

         

 

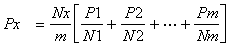
7.1.4 Modified Normal Ratio Method

Normal ratio method is modified to incorporate the effect of distance in the estimation of missing rainfall.

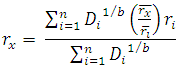   (7.3)

Where  is normal rainfall,  is the distance between the index station i and the gauge station with missing data or ungaged station, n is the number of index stations and b is the constant by which the distance is weighted (normally 1.5-2.0) commonly used D^0.5

 

7.1.5Inverse Distance Method

The inverse distance method has been advocated to be the most accurate method as compare to other two methods discussed above.

Amount of rainfall to be estimated at a location is a function of;

1. rainfall measured at the surrounding index stations

2. distance to each index station from the ungauged location

 

Rainfall r_x at station x is given by;

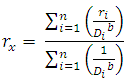(7.4)

b = 2 is commonly used.

As in inverse distance method the weighting is strictly based on distance, hence this method is not satisfactory for hilly regions.

 

Example 7.2 Data for the base station and 5 surrounding stations are tabulated below. Find missing data at ‘A’ using (i) modified normal ratio method and (ii) inverse distance method.

Station	Distance (D) from the base station (km)	Rainfall (cm|)	Normal Rainfall (cm)
A (base station)	-	?	102
B	1.5	2.5	114
C	1.21	3.4	122
D	0.85	1.5	95
E	1.3	2.2	106
F	2.11	1.8	104

 

Solution: (i) Using modified normal ratio method

Weight calculation for different stations other than the base station is shown in following table, using b = 2

Station		Weight (a)
B	1.22
C	1.1
D	0.92
E	1.14
F	1.45
Sum	5.83	1.0

 

Hence,

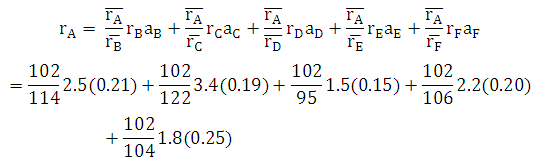
  = 2.12 cm

(ii) Using Inverse Distance Method

Station		Weight (a)
B	0.44
C	0.68
D	1.38
E	0.59
F	0.22
Sum	3.31	1.0

 

Hence,

         

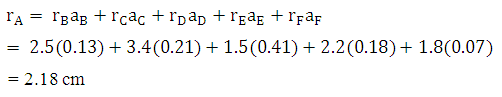
7.1.6Linear Programming Method

Linear programming (LP) method selects a base station and several surrounding index stations and determines optimal weighting factor by minimizing the deviation between observed and computed rainfall at a base station for a number of rainfall events.

 

Thus it determines optimal weighting factors for the base station and associated index stations.

 

This method can be formulated as,Objective is to         minimize sum of deviation for k events i.e., Minimize 

Subjected to     

                                           

                            

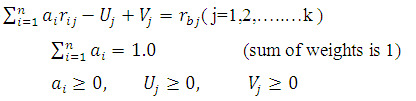
(Non-negativity constraints)

Where,        i = index for “index station”

                   j = index for rainfall events

                    = observed rainfall at base station ‘b’ for event ‘j’

           = computed rainfall at base station for event ‘j’

 

For any event, computed rain – observed rain = deviation

Deviation could be either positive or negative value (unrestricted in sign), such variables are replaced by the difference of two non-negative variables (LP requirement) i.e., U.

 

Solved Example

Assume that rainfall is not known at the station D. The normal precipitations of the three neighbouring gauging stations are as follows:

Station	Station co-ordinate	Normal Annual Precipitation (cm)	Precipitation (cm)
A	(1,2.5)	28	25
B	(4,1)	15	10
C	(3,5)	30	25
D	(3,3)	25	?

 

Compute the rainfall at this point using

a.  Simple Arithmetic Method

b.  Normal Ratio Method

c.   Modified Normal Ratio Method

d.  Inverse Distance Method

 

Answer

a.  Simple Arithmetic Method

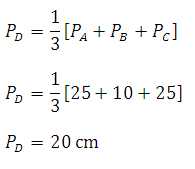
 b.  Normal Ratio Method

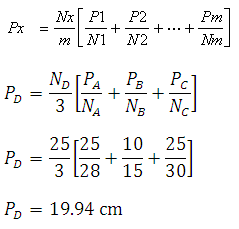
 c.   Modified Normal Ratio Method

 

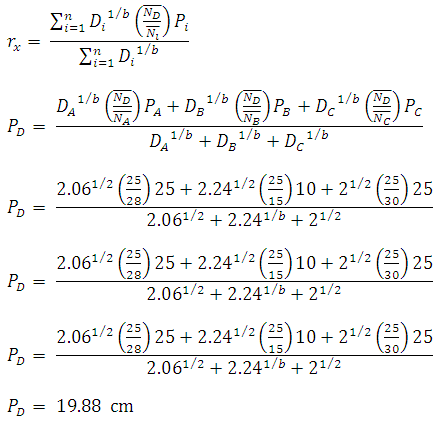
 d.  Inverse Distance Method

 

 

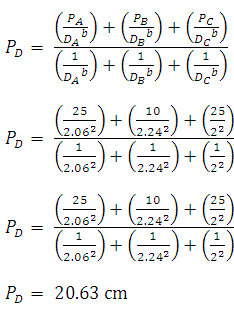
References

Singh, V. P. (1994). Elementary Hydrology.Prentice Hall of India Private Limited,New Delhi.

 

Suggested Reading

Subramanya, K. (1994). Engineering Hydrology.Third edition, Tata McGraw Hill, New Delhi.