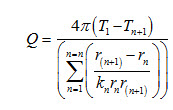Heat and Mass Transfer 2(2+0)

Lesson 3. One dimensional steady state conduction through plane and composite walls, tubes and spheres without heat generation

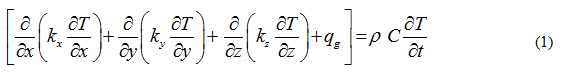
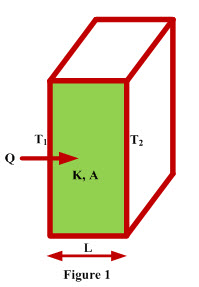
Since it is a case of one-dimensional, stead heat conduction through a wall of uniform conductivity without heat generation, therefore, 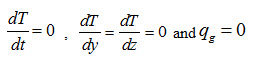  and

Therefore, equation (1) reduces to

Equation (2) is used to determine the temperature distribution and heat transfer rate through the wall. Integrating equation (2) twice with respect to x, it can written as

T = C₁ x + C₂                                                                                                                                                 (3)

Where, C₁ and C₂ are constants of integration.

Using the following boundary conditions:

i.      At x = 0, T = T₁

        Equation (3) is written as C₂ =   T₁                      (4)

ii.      At x = L, T = T₂

Equation (3) can be written as T₂ = C₁ L + C₂

Or         T₂ = C₁ L + T₁

C₁= (T₂ – T₁)/L                                                                                              (5)

Substituting the values of C₁ and C₂ in equation (3)

Equation (6) represents temperature distribution in the wall. It means temperature at any point along the thickness of the wall can be obtained if values of temperatures T₁, T₂, thickness L and distance of the point form either of the faces of the wall are known.

Rate of heat transfer can be determined by using Fourier’s law and can be expressed as

Integrating equation (6) with respect to x to obtain the expression for temperature gradient

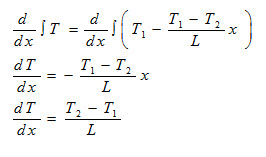
Substituting the value of  from above equation in equation (7), we get

Equation (8) represents the heat transfer rate through the wall.     

ii) Cylinder of Uniform Conductivity without Heat Generation:

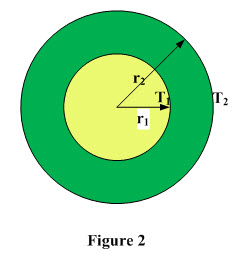
Consider steady state heat conduction through a cylinder having r₁ and r₂ as inner and outer radii respectively and length ‘L’ as shown in Figure 2. Temperature of the inner and outer surfaces is T₁ and T₂ respectively. Heat is flowing from inner to outer surface as T₁ is greater than T₂. The general conduction equation which governs the conduction heat transfer is written as

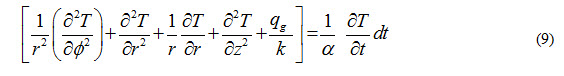
Since it is a case of one-dimensional, stead heat conduction through a wall of uniform conductivity without heat generation, therefore,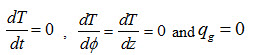

Therefore, equation (9) reduces to

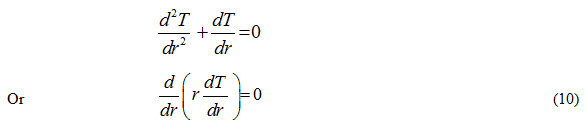
Equation (10) is used to determine the temperature distribution and heat transfer rate through the cylinder. Integrating equation (10) twice with respect to r, it can written as

 and       T = C₁ log_e r + C₂                                                                                                                                              (12)

Using the following boundary conditions:

i. At r = r₁, T = T₁

Equation (12) is written as T₁ = C₁ log_e r₁ + C₂                      (13)

ii. At r = r₂, T = T₂

Equation (12) can be written as

T₂ = C₁ log_e r₂ + C₂                           (14)

 Subtracting equation (14) from equation (13), we get

T₁ – T₂ = C₁ log_e r_1­ – C₁ log_e r₂                         

                                   

Substituting the values of C₁ from equation (15) in equation (13)

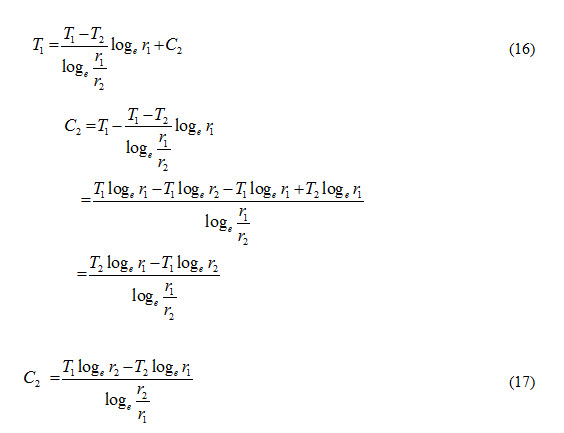
Substituting the values of C₁ and C₂ in equation (12), we get

                    

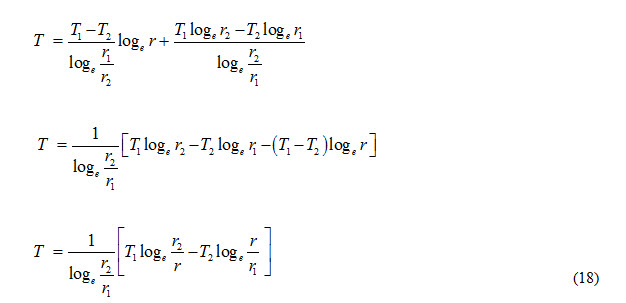
Equation (18) represents temperature distribution in the cylinder. Rate of heat transfer can be determined by using Fourier’s law and can be expressed as

From equation (11) we can write

Substituting the value of C₁ from equation (15), we can write

Substituting the value of from equation (21) in equation (20), we get

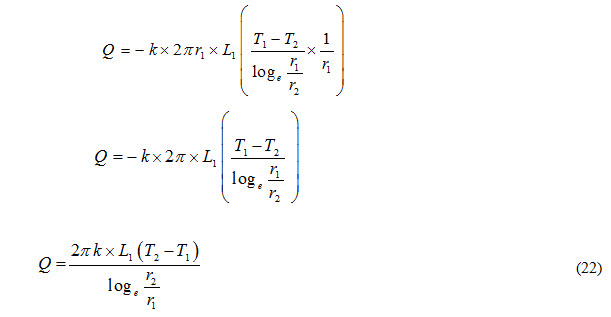
Equation (22) represents the heat transfer rate through the cylinder. 

 iii) Sphere of Uniform Conductivity without Heat Generation:

Consider steady state heat conduction through a hollow sphere having r₁ and r₂ as inner and outer radii respectively. Temperature of the inner and outer surfaces is T₁ and T₂ respectively. Heat is flowing from inner to outer surface as T₁ is greater than T₂. The general conduction equation which governs the conduction heat transfer is written as

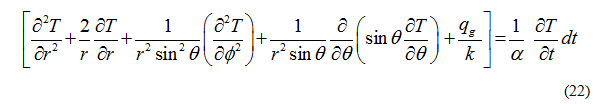
Since it is a case of one-dimensional, steady heat conduction through a shere without heat generation, therefore,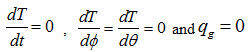

Therefore, equation (22) reduces to

Multiplying both sides of equation (23) by r² , we get

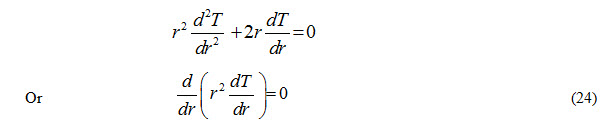
Equation (24) is used to determine the temperature distribution and heat transfer rate through the wall. Integrating equation (23) twice with respect to r, it can written as

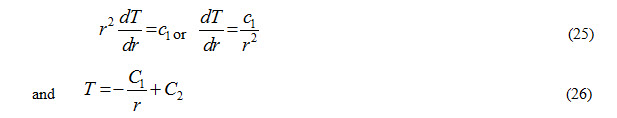
Using the following boundary conditions:

i. At r = r₁, T = T₁

Equation (26) is written as

ii. At r = r₂, T = T₂

Equation (26) can be written as

Subtracting equation (28) from equation (27), we get

Substituting the values of C₁ from equation (29) in equation (27)                                                                           

Substituting the values of C₁ and C₂ from equations (29) and (30) in equation (26)

                              

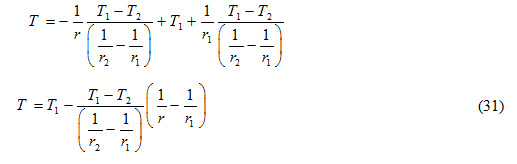
Equation (31) represents temperature distribution in a sphere. Rate of heat transfer can be determined by using Fourier’s law and can be expressed as

From equation (25) we can write

Substituting the value of C₁ from equation (29), we can write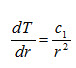

                                                                         

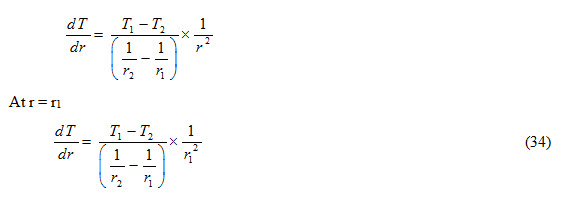
Substituting the value of  from equation (34) from equation (33), we get

                                                      

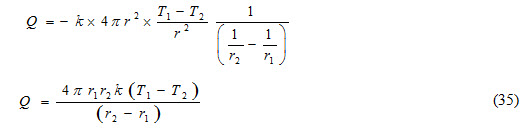
Equation (35) represents the heat transfer rate through a sphere. 

Heat Flow through Composite Geometries:

A) Composite Slab or Wall:

Consider a composite slab made of three different materials having conductivity k₁, k₂ and k₃, length L₁, L₂ and L₃   as shown in Figure 3. One side of the wall is exposed to a hot fluid having temperature T_f and on the other side is atmospheric air at temperature T_a. Convective heat transfer coefficient between the hot fluid and inside surface of wall is h_i (inside convective heat transfer coefficient) and h_o is the convective heat transfer coefficient between atmospheric air and outside surface of the wall (outside convective heat transfer coefficient). Temperatures at inner and outer surfaces of the composite wall are T₁ and T₄ whereas at the interface of the constituent materials of the slab are T₂ and T₃ respectively.

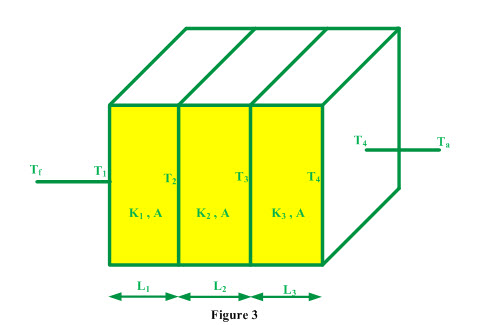
Heat is transferred from hot fluid to atmospheric air and involves following steps:

i)        Heat transfer from hot fluid to inside surface of the composite wall by convection

ii)      Heat transfer from inside surface to first interface by conduction

iii)    Heat transfer from first interface to second interface by conduction

iv)    Heat transfer from second interface to outer surface of the composite wall by conduction

v)      Heat transfer from outer surface of composite wall to atmospheric air by convection

Adding equations (36), (37), (38) and (40), we get

 

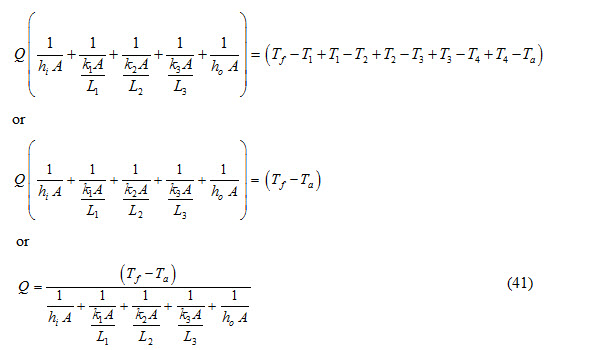
  If composite slab is made of ‘n’ number of materials, then equation (41) reduces to

If inside and outside convective heat transfer coefficients are not to be considered, then equation (42) is expressed as

B) Composite Cylinder:

Consider a composite cylinder consisting of inner and outer cylinders of radii r₁, r₂ and thermal conductivity k₁, k₂ respectively as shown in Figure 4. Length of the composite cylinder is L. Hot fluid at temperature T_f is flowing inside the composite cylinder. Temperature at the inner surface of the composite cylinder exposed to hot fluid is T₁ and outer surface of the composite cylinder is at temperature T₃ and is exposed to atmospheric air at temperature T_a. The interface temperature of the composite cylinder is T₂._. Convective heat transfer coefficient between the hot fluid and inside surface of composite cylinder is h_i (inside convective heat transfer coefficient) and h_o is the convective heat transfer coefficient between atmospheric air and outside surface of the composite cylinder (outside convective heat transfer coefficient). Heat is transferred from hot fluid to atmospheric air and involves following steps:

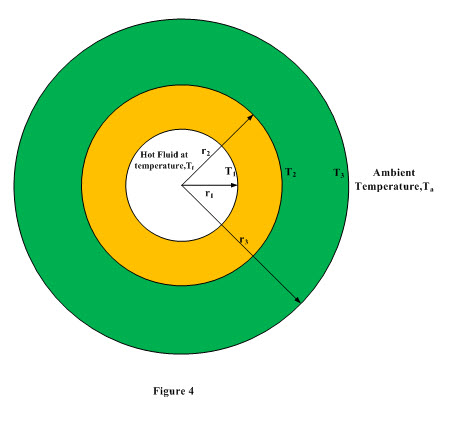
i)                   Heat transfer from hot fluid to inside surface of the composite cylinder by convection

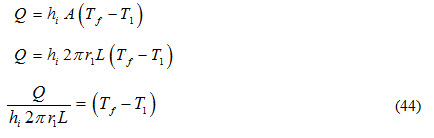
ii)                  Heat transfer from inside surface to interface by conduction

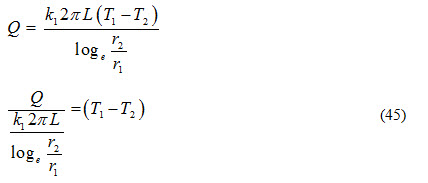
iii)                Heat transfer from interface to outer surface of the composite cylinder by conduction

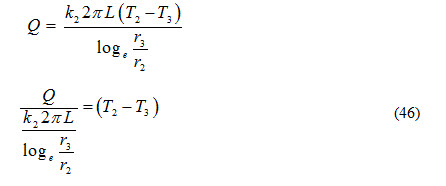
iv)                Heat transfer from outer surface of composite wall to atmospheric air by convection

Adding both sides of equations (44), (45),(46) and (47), we get

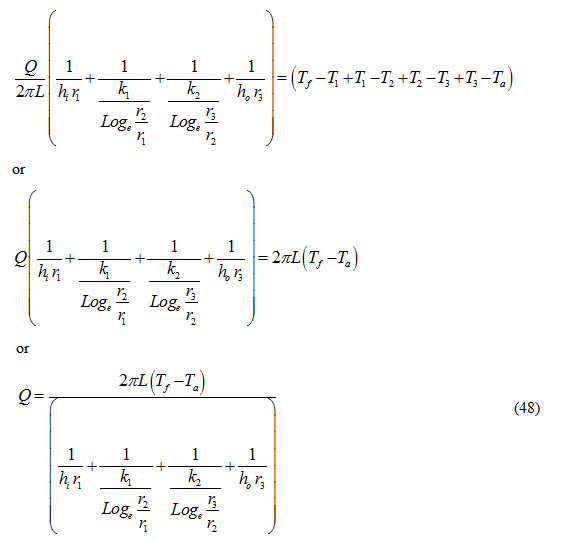
  If the composite cylinder consists of ‘n’ cylinders, then equation (48) can be expressed as:

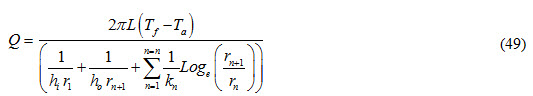
If inside and outside convective heat transfer coefficients are not to be considered, then equation (3.41) is expressed as

C) Composite Sphere:

Consider a composite sphere consisting of inner and outer cylinders of radii r₁, r₂ and thermal conductivity k₁, k₂ respectively. Hot fluid at temperature T_f is flowing inside the composite sphere. Temperature at the inner surface of the composite sphere exposed to hot fluid if T₁ and outer surface of the composite cylinder is at temperature T₃ and is exposed to atmospheric air at temperature T_a. The interface temperature of the composite cylinder is T₂. Convective heat transfer coefficient between the hot fluid and inside surface of composite sphere is h_i (inside convective heat transfer coefficient) and h_o is the convective heat transfer coefficient between atmospheric air and outside surface of the composite sphere (outside convective heat transfer coefficient). Heat is transferred from hot fluid to atmospheric air and involves following steps:

i)              Heat transfer from hot fluid to inside surface of the composite sphere by convection

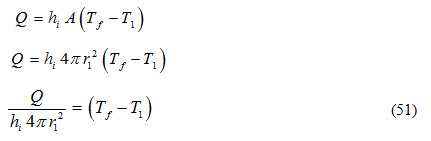
ii)            Heat transfer from inside surface to interface by conduction.

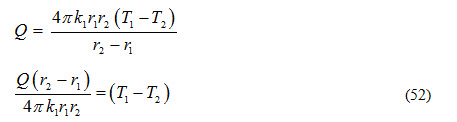
iii)          Heat transfer from interface to outer surface of the composite sphere by conduction

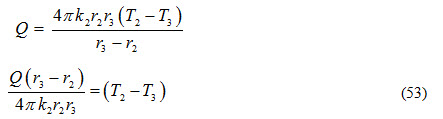
iv)             Heat transfer from outer surface of composite wall to atmospheric air by convection

Adding both sides of equations (51), (52),(53) and (54), we get

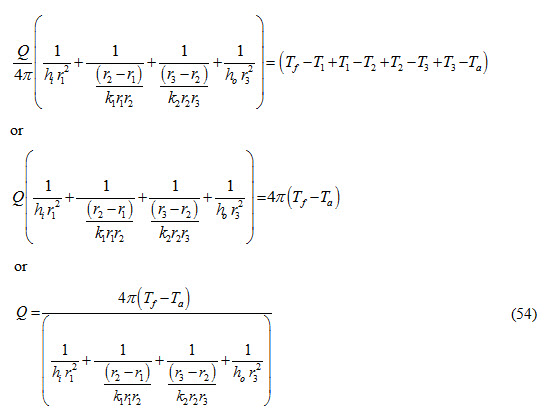
  If the composite sphere consists of ‘n’ concentric spheres, then equation (54) can be expressed as:

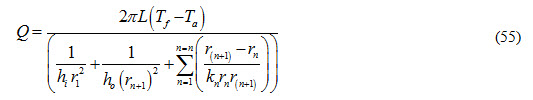
If inside and outside convective heat transfer coefficients are not to be considered, then equation (55) is expressed as