Heat and Mass Transfer 2(2+0)

Lesson 11. Fin Efficiency and effectiveness, Problems on fins

Free Convection:

If motion of heated fluid particles occurs due to density differences resulting from temperature variation, heat transfer process is termed as free convection. Heating of a liquid in a vessel placed on a stove is an example of free convection heat transfer process as shown in Figure 1.   

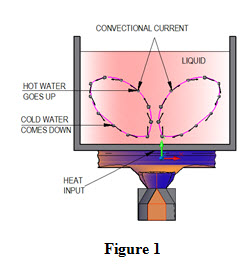
Forced Convection:

If motion of heated fluid particles is caused by some external source such as a fan, blower or a pump, then heat transfer process is termed as forced convection. The heating of air by blowing it over electric coils in a heater and cooling of air by blowing it over cooling coils in an air conditioner are examples of forced convection as shown in Figure 2.

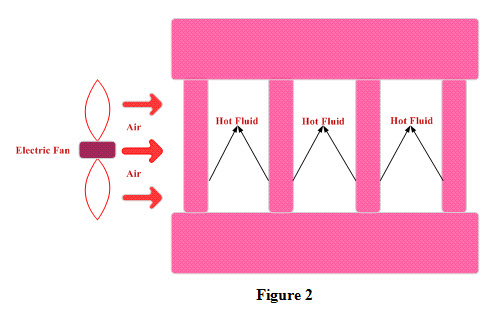
Newton’s Law of Cooling:

During study of cooling of fluids, Newton observed that convection process plays a more prominent role when a fluid and a solid surface exchange heat as compared to pure conduction. He proposed fundamental heat convection equation which is expressed as

q = h A (T_s – T_f)                                                                             (1)

 where

             q is average rate of heat transfer by convection, W or J/sec

            A is heat transferring area, m²

            T_s is temperature of solid surface, ^oC

            T_f is temperature of fluid, ^oC

            h is an average value of proportionality constant called convective heat transfer

            coefficient, W/(m²-^oC)

Convective heat transfer coefficient, h:

It is not a fluid property but its value depends upon the following parameters

i.      Surface geometry

ii.      Nature of fluid flow

iii.      Bulk fluid velocity

iv.      Thermo-physical properties such as thermal density, specific heat, viscosity and thermal conductivity of fluid

Typical values of convective heat transfer coefficient are given in Table 1.

Table 1: Value of Convective Heat Transfer Coefficient ( W/(m²-K))

Type of Convection	Gases	Liquids
Free Convection	2-25	10-1000
Forced Convection	25-250	50-20,000
Boiling and Condensation	2500-10000

 

Non- Dimensional Numbers:

i)     Reynolds Number: Reynolds number is a measure of relative magnitude of inertia force to viscous force occurring in a flow. Higher is the value of Reynolds number, greater will be inertia force and vice-versa. Reynolds number is defined as the ratio of inertia force to viscous force and is expressed as

ii)      Prandtl Number: It is defined as the ratio of kinematic viscosity to thermal diffusivity and is denoted by ‘Pr’.

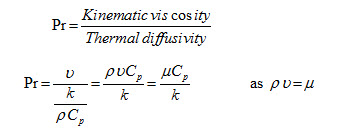
Kinematic viscosity indicates impulse transport through molecular friction and thermal diffusivity indicates heat transport through conduction. Prandtl number signifies the relative speed with which momentum and energy are propagated through a fluid.

iii)    Nusselt Number: It is defined as the ratio of heat flow rate by convection under unit temperature gradient to the heat flow rate by conduction under unit temperature gradient through a stationary thickness ‘L’.

      

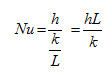
iv)    Stanton Number: Stanton number is defined as ratio of Nusselt number to the product of Reynolds and Prandtl numbers and is denoted by ‘St’.

v)      Peclet Number: Peclet number is defined as ratio of mass heat flow rate to heat flow rate by conduction under unit temperature gradient through a thickness of ‘L’.

Mass heat flow rate = Heat capacity of fluid passing through a unit cross-sectional area per unit time

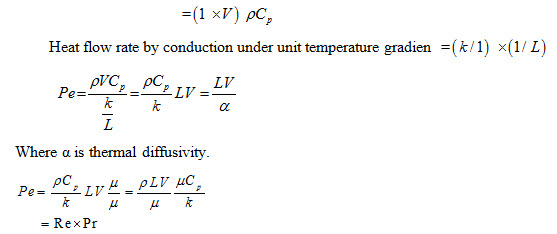
vi)    Gratez Number: It is defined as the ratio of heat capacity of a fluid flowing through a pipe per unit length to the conductivity of the pipe material.

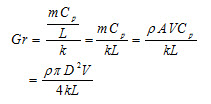
vii)  Grashoff Number: It is defined as the ratio of product of inertia force and buoyancy force to square of viscous force.