LESSON 7.

Introduction

In all the examples considered in the previous module, the equations of static equilibrium (section 1.6) alone were sufficient to determine the unknown support reactions and internal forces. Such structures are called determinate structures. However in many practical structures, the number of unknown may exceed the number of equilibrium conditions and therefore the equations of statics alone cannot provide the solution. Such structures are called statically indeterminate or redundant structures. The extra unknowns are due to more number of external supports or internal members or both than that of actually required to maintain the static equilibrium configuration. In order to analyze indeterminate structures, additional equations considering the geometry of the deflected shape, also known as compatibility equations are required. In this lesson and the subsequent lessons in this module we will learn several methods to analyze statically indeterminate beams and rigidly jointed frames.

7.1 Static Indeterminacy

The degree of static indeterminacy or redundancy is defined as,

Degree of static indeterminacy = Total number of unknown (external and internal) - Number of independent equations of equilibrium

For instance, in the cantilever beam shown in Figure 7.1, the number of unknown reactions is three, viz, Ax, Ay and MA. These three unknowns can be solved by three static equilibrium equations \[\sum {{F_x}}=0\] , \[\sum {{F_y}}=0\] , \[\sum {{M_A}}=0\]   and therefore this is a determinate structure. Now if end B is propped as shown in Figure 7.2, the number of unknown reactions becomes four (Ax, Ay, MA and By) while the total number equations remain as three. Hence the beam now becomes statically indeterminate with degree of indeterminacy one. 

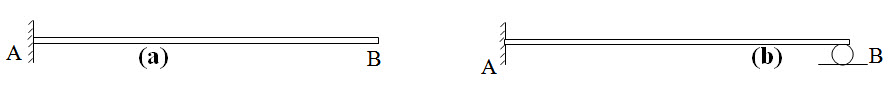
Module 2 Lesson 7 Fig.7.1

Fig.7.1.

For statically indeterminate beams and rigidly jointed frames, there are two types of indeterminacy, i) external indeterminacy and ii) internal indeterminacy.

7.1.1 External Indeterminacy

The external indeterminacy is the excess of total number of support reactions over the static equilibrium equations. Some examples are given below,

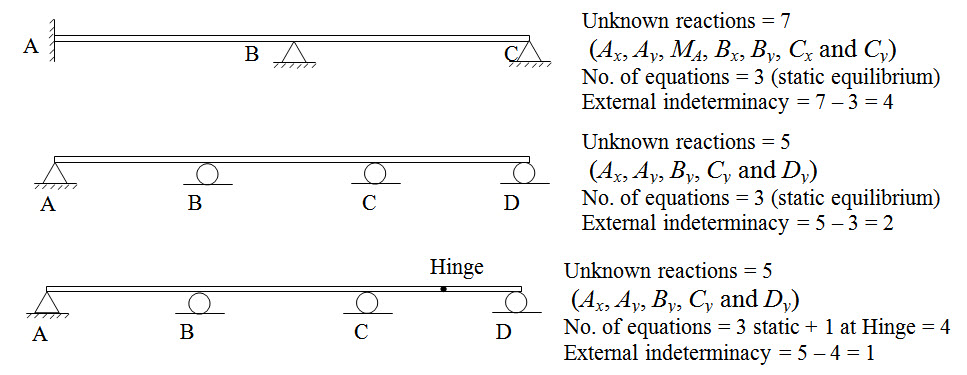
Module 2 Lesson 7 Fig.7.2

Fig. 7.2.

In the case of continuous beam shown above the internal forces (shear and moment) at any point in the beam can be determined by static equilibrium equations once the support reactions are known. Therefore, these beams are determinate internally but indeterminate externally.

7.1.2 Internal indeterminacy

Structures may also become indeterminate due to more number of members than that of actually required to maintain the static equilibrium configuration. Unlike externally indeterminate structures, here internal member forces may not be determined by static equilibrium equations even though the support reactions are known and therefore these structures are called internally indeterminate structures.

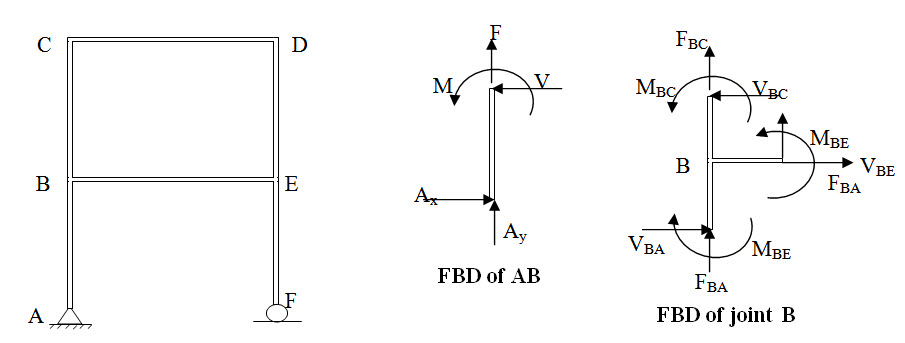
Module 2 Lesson 7 Fig.7.3

 Fig. 7.3.

For illustration purpose, consider a rigid frame as shown in Figure 7.3. There are three unknown reactions (Ax, Ay and Fy) which can be determined by three equilibrium equations. Thus the structure is externally determinate. By considering free body diagram of member AB, axial force (F), shear force (V) and moment (M) at any point in AB can be computed. Now consider joint B at which there are total nine internal forces (viz, axial, shear and moment each for BA, BC and BE). Since internal forces in member BA are already known, the number of unknown at B is six. Similarly by taking FBD of any joint one can see that the number of unknown internal forces is always six. As only three equilibrium equations are available, here internal indeterminacy is three.

In general the degree of internal indeterminacy of rigid frames is represented by,

\[I=3a\]

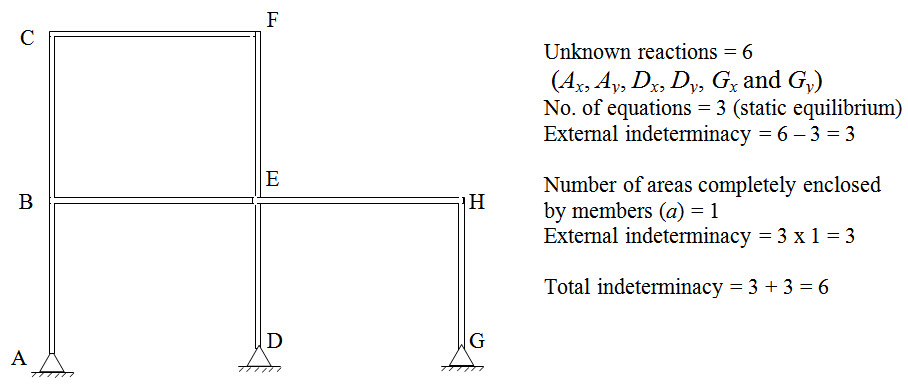
where, a is the number of areas completely enclosed by members of the frame. For the rigid frame shown in Figure 7.3, a = 1 and therefore degree of internal indeterminacy is \[3 \times 1=3\].

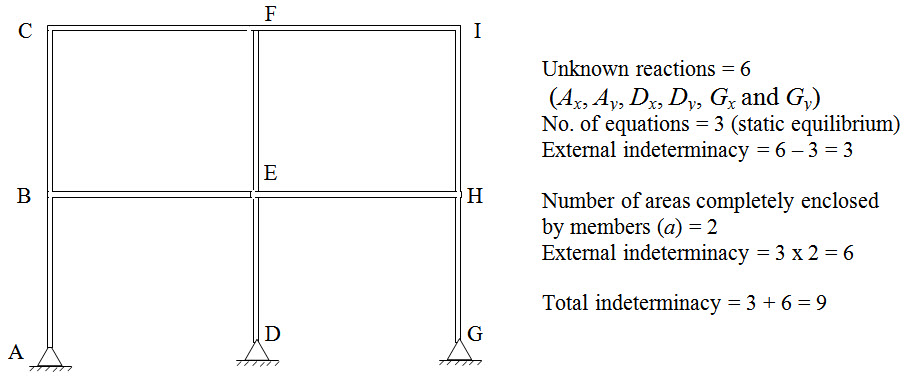
7.1.3 Total Indeterminacy

Total indeterminacy = external indeterminacy + internal indeterminacy

Some examples are given bellow,

Module 2 Lesson 7 Fig.7.1.3 Module 2 Lesson 7 Fig.7.1.3_2

7.2 Method of Analysis of Statically Indeterminate Beams

The method of analysis of statically indeterminate beams may broadly be classified into two groups; (i) force method or flexibility method, and (ii) displacement method or stiffness method.

  • Force method: In this method the redundant forces are taken as unknowns. Additional equations are obtained by considering displacement compatibility.

  • Displacement method: In this method, displacements of joints are taken as unknowns. A set of algebraic equations in terms of unknown displacements is obtained by substituting the force-displacement relations into the equilibrium equations.

The choice between the force method and the displacement method mainly depends upon the type of structure and the support conditions. However these two methods are just the alternate procedure to solve the same basic equations.

 

Suggested Readings

Hbbeler, R. C. (2002). Structural Analysis, Pearson Education (Singapore) Pte. Ltd.,Delhi.

Jain, A.K., Punmia, B.C., Jain, A.K., (2004). Theory of Structures. Twelfth Edition, Laxmi Publications. 

Menon, D.,  (2008), Structural Analysis, Narosa Publishing House Pvt. Ltd., New Delhi.

Hsieh, Y.Y., (1987), Elementry Theory of Structures , Third Ddition, Prentrice Hall.

Last modified: Monday, 23 September 2013, 5:42 AM