Strength of Material 3(2+1)

LESSON 15. Displacement Method: Moment Distribution Method – 1

Sign Convention

For the development and application of Moment Dsitribution Method we will use similar sign convention as in the case of Slope-Deflection Method.

Moment: At the end of a member clockwise moment is positive.

Transverse displacement: Transverse displacement in upward direction is positive.

Rotation: Rotation in anti-clockwise direction is positive.

The above sign convention is depicted in Figure 15.1.

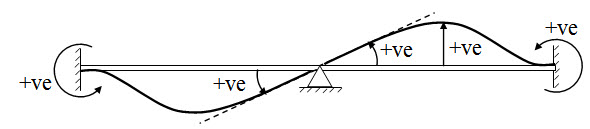
Fig. 15.1.

15.1 Absolute and Relative Stiffness

Stiffness of a member may be defined as the force/moment required to cause unit displacement/rotation. The central idea of Moment distribution method is to distribute moment at any joint, among the connenting members (members meeting at that joint) according to their rotational stiffnesses. In this section we will derive the expressions of rotational stifness of member with different support conditions.

15.1.1 Beam Hinged at Both Ends

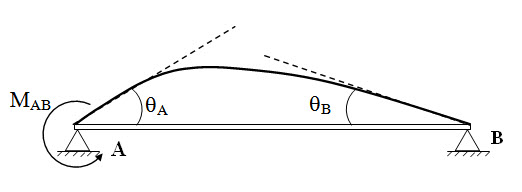
Fig. 15.2.

Slope deflection equation at A and B (δ = 0),

\[{M_{AB}} = {{2EI} \over L}\left( {2{\theta _A} + {\theta _B}} \right)\]                                                                             (15.1)

       \[{M_{BA}} = {{2EI} \over L}\left( {{\theta _A} + 2{\theta _B}} \right)\]                                                                             (15.2)

Now, at B, equilibrium equation is,M_BA = 0 . Therefore form equaition (2), we have,

\[{\theta _B}=-{1 \over 2}{\theta _A}\] .

Substituting, θ_B = -θ_A/2   in equation (1), we have,

\[{M_{AB}} = {{2EI} \over L}\left( {2{\theta _A} - {{{\theta _A}} \over 2}} \right)\]

\[\Rightarrow {M_{AB}} = {{3EI} \over L}{\theta _A}\]

Absolute Stiffness \[k = {{3EI} \over L}\]

15.1.2 Beam Hinged at one End and Fixed at other End

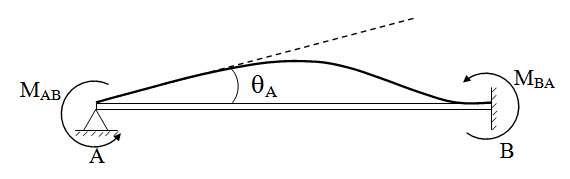
Fig. 15.3.

Slope deflection equation at A and B (q_B = 0, d = 0),

\[{M_{AB}} = {{4EI} \over L}{\theta _A}\]                                                                                             (15.3)

\[{M_{BA}} = {{2EI} \over L}{\theta _A}\]                                                                                             (15.4)

From equations (3) and (4), we have,

\[{M_{BA}} = {1 \over 2}{M_{AB}}\] .                                                                                                   (15.5)

Absolute Stiffness   \[k = {{4EI} \over L}\]

15.1.3 Several members meeting at a joint

 

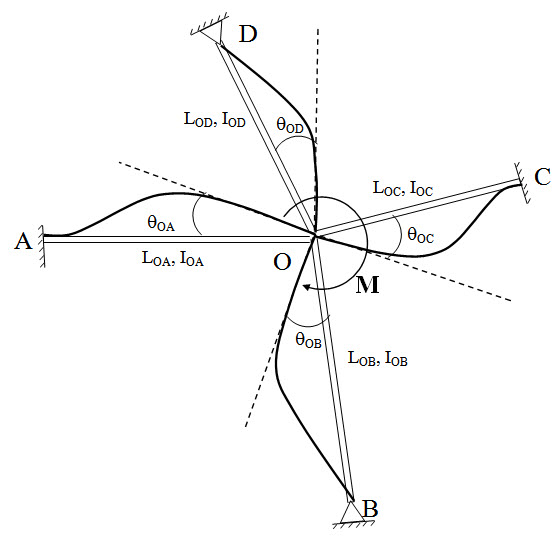
Fig. 15.4.

Figure 15.4 shows, four members (for illustration purpose only four members are taken, but the theory is applicable for any number of members), AO, BO, CO and DO meeting at O. L_OA, L_OB, L_OC, and L_OD are the length and  I_OA, I_OB, I_OC, and I_OD are the second moment of area of the respective members. Support A, C are fixed and B, D are hinged. An external moment M is applied at O. The moment M will be distributed among all the members meeting at O. Suppose M_OA, M_OB, M_OC, and M_OD are the corresponding distribution.

Compatibility condition at O,

\[{\theta _{OA}} = {\theta _{OB}} = {\theta _{OC}} = {\theta _{OD}} = \theta \]                                       (15.6)

Equilibrium condition at O,

\[{M_{OA}} = {M_{OB}} = {M_{OC}} = {M_{OD}} = M\]                                                                         (15.7)

Now from the previous tow cases, we may express M_OA, M_OB, M_OC, and M_OD as,

\[{M_{OA}} = {{4E{I_{OA}}} \over {{L_{OA}}}}\theta={k_{OA}}\theta \]                                            (15.8)

\[{M_{OB}} = {{3E{I_{OB}}} \over {{L_{OB}}}}\theta={k_{OB}}\theta \]                                            (15.9)

From Equations (8a) – (8b),

\[{M_{OA}}:{M_{OB}}:{M_{OC}}:{M_{OD}}::{k_{OA}}:{k_{OB}}:{k_{OC}}:{k_{OD}}\]             (15.30)

From equations (7) and (9),

\[{M_{OA}} = {{{k_{OA}}} \over {{k_{OA}} + {k_{OB}} + {k_{OC}} + {k_{OD}}}}M = {{{k_{OA}}} \over {\sum k }}M\]

\[{M_{OA}} = {{{k_{OB}}} \over {{k_{OA}} + {k_{OB}} + {k_{OC}} + {k_{OD}}}}M = {{{k_{OB}}} \over {\sum k }}M\]

\[{M_{OA}} = {{{k_{OC}}} \over {{k_{OA}} + {k_{OB}} + {k_{OC}} + {k_{OD}}}}M = {{{k_{OC}}} \over {\sum k }}M\]

\[{M_{OA}} = {{{k_{OD}}} \over {{k_{OA}} + {k_{OB}} + {k_{OC}} + {k_{OD}}}}M = {{{k_{OD}}} \over {\sum k }}M\]

Therefore, moment acting at a joint will be divided amongst the connecting members in proportion to their stiffness.

The factors  \[{{{k_{OA}}} \over {\sum k }}\] , \[{{{k_{OB}}} \over {\sum k }}\] ,  \[{{{k_{OC}}} \over {\sum k }}\]  ,  and \[{{{k_{OD}}} \over {\sum k }}\] are called distribution factor (DF) and moments \[{M_{OA}}\] ,  \[{M_{OB}}\] ,  \[{M_{OC}}\] ,  and \[{M_{OD}}\]  are called distributed moments.

15.1.4 Carry Over Factor

Consider a fixed beam AB as shown bellow. Suppose the rotational constraint of joint A is released and a balancing moment M_AB is applied at A. Then M_AB will cause a moment M_BA at B.

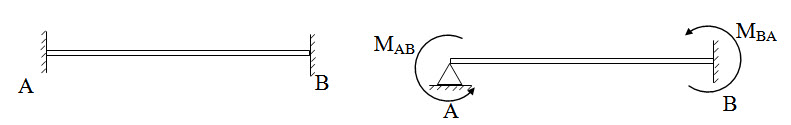
 Fig. 15.5.

The carry over factor is defined as,

\[{C_{AB}} = {{{M_{BA}}} \over {{M_{AB}}}}\]

From 15.1.2 we have,

\[{C_{AB}} = {{{M_{BA}}} \over {{M_{AB}}}} = {1 \over 2}\]

Here, \[{M_{BA}}\]  is called caried over momnet at B due to \[{M_{AB}}\]  at A.

 

Suggested Readings

Hbbeler, R. C. (2002). Structural Analysis, Pearson Education (Singapore) Pte. Ltd.,Delhi.

Jain, A.K., Punmia, B.C., Jain, A.K., (2004). Theory of Structures. Twelfth Edition, Laxmi Publications. 

Menon, D.,  (2008), Structural Analysis, Narosa Publishing House Pvt. Ltd., New Delhi.

Hsieh, Y.Y., (1987), Elementry Theory of Structures , Third Ddition, Prentrice Hall.